Knowing the units of the rate constant is important as it is used often for solving problems related to the rate laws.

 

k Units of a Zero-Order Reaction

Zero-order indicates that the rate does not depend on the concentration, and therefore, the rate is equal to the concentration.

rate = k[A]⁰

[A]⁰ = 1, therefore,

rate = k

The units for the rate are mol/L, so it is the same as the rate constant:

k = mol/L s or M/s or M x s^-1

 

k Units of a First-Order Reaction

Let’s assume it is a first-order reaction in molecule A:

rate = k[A]

The units for the rate are mol/L. The rate constant is equal to:

 

\[k\; = \;\frac{{{\rm{rate}}}}{{\left[ {\rm{A}} \right]}}\]

 

And now, add the units for the rate and concentration:

 

\[k\; = \;\frac{{{\rm{mol}}}}{{{\rm{L}}\; \times \;{\rm{s}}}}\; \div \;\frac{{{\rm{mol}}}}{{{\rm{L}}\;}}\; = \;\frac{{\cancel{{{\rm{mol}}}}}}{{\cancel{{\rm{L}}}\; \times \;{\rm{s}}}}\; \times \;\frac{{\cancel{{\rm{L}}}}}{{\cancel{{{\rm{mol}}}}}}\; = \;{{\rm{s}}^{{\rm{ – 1}}}}\]

 

k Units of a Second-Order Reaction

Let’s assume it is a second-order reaction in molecule A:

 

rate = k[A]²

 

\[k\; = \;\frac{{{\rm{rate}}}}{{{{\left[ {\rm{A}} \right]}^2}}}\]

 

And now, add the units for the rate and concentration:

 

\[k\; = \;\frac{{{\rm{mol}}}}{{{\rm{L}}\; \times \;{\rm{s}}}}\; \div \;{\left( {\frac{{{\rm{mol}}}}{{{\rm{L}}\;}}} \right)^2}\; = \;\frac{{\cancel{{{\rm{mol}}}}}}{{\cancel{{\rm{L}}}\;{\rm{ \times }}\;{\rm{s}}}}\;{\rm{ \times }}\;\frac{{{{\rm{L}}^{\cancel{{\rm{2}}}}}}}{{{\rm{mol}}\cancel{{^{\rm{2}}}}}}\;\;{\rm{ = }}\;\frac{{\rm{L}}}{{{\rm{mol}}\; \times \;{\rm{s}}}}\]

 

Alternatively, this can be written as M^-1 · s^-1

 

A Shortcut to Determining the Units of Rate Constant

Notice how for each order, we determined the units of k by dividing the rate by molarity raised to the power of the reaction order:

 

\[k\; = \;\frac{{{\rm{rate}}}}{{{{\left[ {\rm{A}} \right]}^n}}}\]

where n is the reaction order

From this equation, a general formula for the units of k is obtained which is:

 

k units = M^1-n · t^-1

where n is the reaction order

For example, let’s say we want to determine the units of the rate constant for third-order reactions. 

n = 3, and therefore, 

k units = M^1-3 · t^-1 = M^-2 · t^-1

 

If the time is seconds, then the units will be:

 

k units = M^-2 · s^-1

 

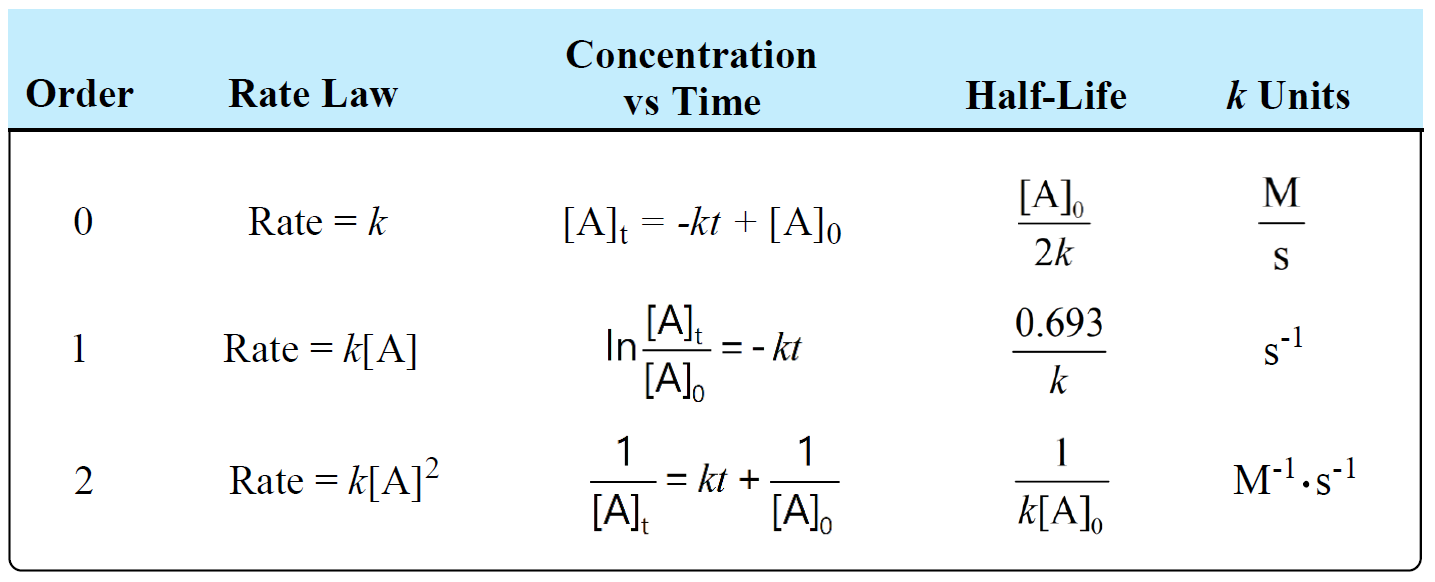
The following table summarizes the rate laws, half-lives, and k units for first-, second-, and zero-order reactions:

 

 

Here is a 77-question, Multiple-Choice Quiz on Chemical Kinetics:

 

Check Also

• Reaction Rate
• Rate Law and Reaction Order
• How to Determine the Reaction Order
• Integrated Rate Law
• The Half-Life of a Reaction
• Determining the Reaction Order Using Graphs
• How Are Integrated Rate Laws Obtained
• Activation Energy
• The Arrhenius Equation
• Chemical Kinetics Practice Problems