$referrer_host = www.google.com. How To Convert Grams To Number of Atoms - Chemistry Steps
Converting atoms to grams factors

Atoms, Molecules, and Ions

In the previous two posts, we discussed converting grams to moles and grams to molecules. For the moles, here is what we do: first look up the molar mass in the periodic table, set up the correct conversion factor, and finally do the multiplication. Here is a short summary for converting the mass to moles:

 

 

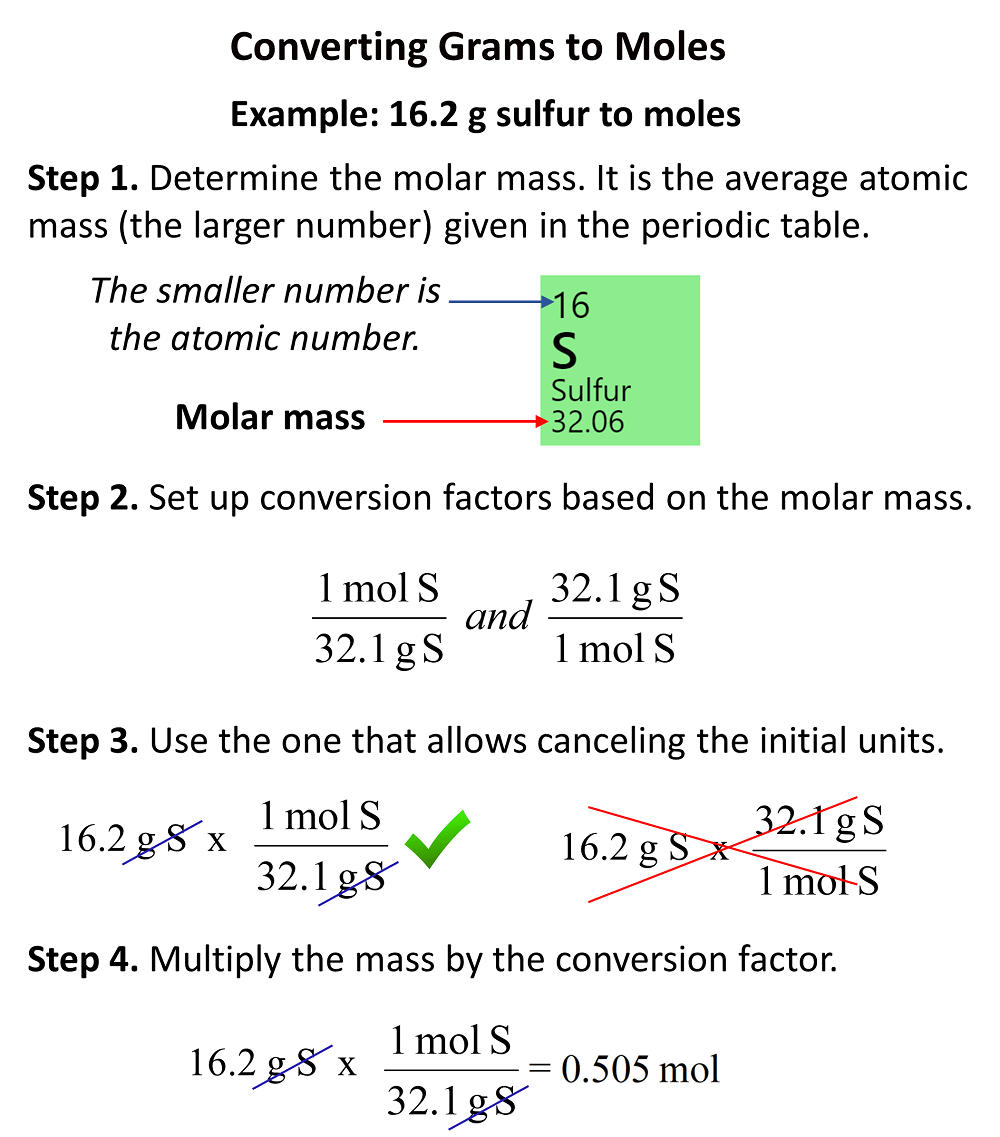
Notice that the exact answer in the calculator is 0.5046728 and we round it off to three significant figures because both initial numbers contain three significant figures. 

To calculate the number of molecules or atoms from mass, we need one extra step using the Avogadro’s number (6.02 x 1023). Remember, the Avogadro’s number shows how many particles, which can be atoms, molecules, or ions, there are in one mole of a sample and because it is related to moles, we need to first convert the mass to moles.

So, there are two steps combined in this conversion and the plan is to first convert the mass to moles and then to the number of atoms using NA:

For example, how many atoms of sulfur are there in its 5.20 g sample?

Just like in the summary example, we are first going to determine the number of moles. The molar mass of sulfur is 32.1 g/mol, and therefore, the conversion factors are:

                                                                               

\[\frac{{{\rm{1}}\;{\rm{mol}}\;{\rm{S}}}}{{{\rm{32}}{\rm{.1}}\;{\rm{g}}\,{\rm{S}}}}\;\,and\,\,\frac{{{\rm{32}}{\rm{.1}}\;{\rm{g}}\,{\rm{S}}}}{{{\rm{1}}\;{\rm{mol}}\;{\rm{S}}}}\]

 

These two simply indicate that 1 mol of S weighs 32.1 g, which is the molar mass of the sulfur which we find in the periodic table.

Now, to calculate the number of moles, we need to choose the correct conversion factor. Remember, the idea is to choose the one that allows canceling the gram units. So, it should be the one where the grams are in the denominator.

 

\[{\rm{n}}\,{\rm{(S)}}\,{\rm{ = }}\,{\rm{5}}{\rm{.20}}\,{\rm{g}}\,{\rm{ \times }}\,\frac{{{\rm{1}}\;{\rm{mol}}\;{\rm{S}}}}{{{\rm{32}}{\rm{.1}}\;{\rm{g}}\,{\rm{S}}}}\;{\rm{ = }}\,{\rm{0}}{\rm{.162}}\,{\rm{mol}}\]

 

Once we have the number of moles, we use another conversion factor linking it with the Avogadro’s number.

 

\[\frac{{{\rm{1}}\;{\rm{mol}}\;{\rm{S}}}}{{{\rm{6}}{\rm{.03}}\;{\rm{ \times }}\,{\rm{1}}{{\rm{0}}^{{\rm{23}}}}\;{\rm{atoms of}}\,{\rm{S}}}}\;{\rm{or}}\,\frac{{{\rm{6}}{\rm{.03}}\;{\rm{ \times }}\,{\rm{1}}{{\rm{0}}^{{\rm{23}}}}\;{\rm{atoms of}}\,{\rm{S}}}}{{{\rm{1}}\;{\rm{mol}}\;{\rm{S}}}}\]

 

And this time, we need to pick the one that allows canceling the mols which is the second conversion factor:

 

\[{\rm{0}}{\rm{.162 }}\cancel{{{\rm{mol}}\;{\rm{S}}}}\, \times \;\frac{{{\rm{6}}{\rm{.02}}\;{\rm{ \times }}\,{\rm{1}}{{\rm{0}}^{{\rm{23}}}}\;{\rm{atoms}}\,{\rm{of}}\,{\rm{S}}}}{{{\rm{1}}\;\cancel{{{\rm{mol}}\;{\rm{S}}}}}}\, = \;9.75\,{\rm{ \times }}\,{\rm{1}}{{\rm{0}}^{{\rm{22}}}}\,{\rm{atoms of}}\,{\rm{S}}\]

 

These conversions can also be combined in one step:

 

\[{\rm{N}}\,{\rm{(S)}}\,{\rm{ = }}\,{\rm{5}}{\rm{.20}}\,\cancel{{{\rm{g}}\,{\rm{S}}}}\;{\rm{ \times }}\,\frac{{{\rm{1}}\;\cancel{{{\rm{mol}}\;{\rm{S}}}}}}{{{\rm{32}}{\rm{.1}}\;\cancel{{{\rm{g}}\,{\rm{S}}}}}}\,{\rm{ \times }}\;\frac{{{\rm{6}}{\rm{.02}}\;{\rm{ \times }}\,{\rm{1}}{{\rm{0}}^{{\rm{23}}}}\;{\rm{atoms}}\,{\rm{of}}\,{\rm{S}}}}{{{\rm{1}}\;\cancel{{{\rm{mol}}\;{\rm{S}}}}}}\,{\rm{ = }}\;{\rm{9}}{\rm{.75}}\,{\rm{ \times }}\,{\rm{1}}{{\rm{0}}^{{\rm{22}}}}\,{\rm{atoms of}}\,{\rm{S}}\]

 

Number of Atoms in a Molecule

Sometimes, you may be asked to calculate the number of atoms for a particular element in a molecule. For example, how many atoms of Cl are there in a 54.0 g sample?

As always, first, we determine the molar mass for calculating the number of moles:

 

M (PCl5) = M (P) + 5 M (Cl) = 31.0 + 5 x 35.5 = 208.5 g/mol

 

The two conversion factors for calculating the moles would be:

 

\[\frac{{{\rm{1}}\;{\rm{mol}}\;{\rm{PC}}{{\rm{l}}_{\rm{5}}}}}{{{\rm{208}}{\rm{.5}}\;{\rm{g}}\,{\rm{PC}}{{\rm{l}}_{\rm{5}}}}}\;{\rm{or}}\,\,\frac{{{\rm{208}}{\rm{.5}}\;{\rm{g}}\,{\rm{PC}}{{\rm{l}}_{\rm{5}}}}}{{{\rm{1}}\;{\rm{mol}}\;{\rm{PC}}{{\rm{l}}_{\rm{5}}}}}\]

 

We are going to use the first conversion factor because it has the units of grams on the denominator which allows us to cancel them with the initial amount given in grams:

 

\[{\rm{n}}\,{\rm{(PC}}{{\rm{l}}_{\rm{5}}}{\rm{)}}\,{\rm{ = }}\,{\rm{54}}{\rm{.0}}\,\cancel{{{\rm{g}}\,{\rm{PC}}{{\rm{l}}_{\rm{5}}}}}\,{\rm{ \times }}\,\frac{{{\rm{1}}\;{\rm{mol}}\;{\rm{PC}}{{\rm{l}}_{\rm{5}}}}}{{{\rm{208}}{\rm{.5}}\;\cancel{{{\rm{g}}\,{\rm{PC}}{{\rm{l}}_{\rm{5}}}}}}}\;{\rm{ = }}\,{\rm{0}}{\rm{.260}}\,{\rm{mol}}\]

 

Once we have the number of moles, we can now use it to calculate the number of molecules using another conversion with the Avogadro’s number.

 

\[\frac{{{\rm{1}}\;{\rm{mol}}\;{\rm{PC}}{{\rm{l}}_{\rm{5}}}}}{{{\rm{6}}{\rm{.02}}\;{\rm{ \times }}\,{\rm{1}}{{\rm{0}}^{{\rm{23}}}}\;{\rm{molecules}}\;{\rm{PC}}{{\rm{l}}_{\rm{5}}}}}\;{\rm{or}}\,\frac{{{\rm{6}}{\rm{.02}}\;{\rm{ \times }}\,{\rm{1}}{{\rm{0}}^{{\rm{23}}}}\;{\rm{molecules}}\,{\rm{PC}}{{\rm{l}}_{\rm{5}}}}}{{{\rm{1}}\;{\rm{mol}}\;{\rm{PC}}{{\rm{l}}_{\rm{5}}}}}\]

 

And this time, we need to pick the one that allows canceling the mols which is the second conversion factor:

 

\[{\rm{0}}{\rm{.26 }}\cancel{{{\rm{mol}}\;{\rm{PC}}{{\rm{l}}_{\rm{5}}}}}\, \times \;\frac{{{\rm{6}}{\rm{.02}}\;{\rm{ \times }}\,{\rm{1}}{{\rm{0}}^{{\rm{23}}}}\;{\rm{molecules}}\,{\rm{PC}}{{\rm{l}}_{\rm{5}}}}}{{{\rm{1}}\;\cancel{{{\rm{mol}}\;{\rm{PC}}{{\rm{l}}_{\rm{5}}}}}}}\, = \;1.57\,{\rm{ \times }}\,{\rm{1}}{{\rm{0}}^{{\rm{23}}}}\,{\rm{molecules PC}}{{\rm{l}}_{\rm{5}}}\]

 

In the last step, we are going to calculate the number of Cl atoms in 1.57 x 1023 molecules of PCl5. For this, we need to understand the formula of PCl5. The subscript 5 indicates that there are 5 chlorine atoms in one molecule of PCl5. Therefore, to determine the number of Cl atoms in 1.57 x 1023 molecules of PCl5, we multiply it by 5:

 

\[{\rm{N}}\,{\rm{(Cl)}}\,{\rm{ = }}\,{\rm{1}}{\rm{.57}}\,{\rm{ \times }}\,{\rm{1}}{{\rm{0}}^{{\rm{23}}}}\,\cancel{{{\rm{molecules}}\;{\rm{of}}\,{\rm{PC}}{{\rm{l}}_{\rm{5}}}}}{\rm{ \times }}\,\frac{{{\rm{5}}\,{\rm{atoms}}\,{\rm{of Cl}}}}{{{\rm{1}}\;\cancel{{{\rm{molecule}}\;{\rm{of}}\;{\rm{PC}}{{\rm{l}}_{\rm{5}}}}}}}\;{\rm{ = }}\;{\rm{7}}{\rm{.85}}\,{\rm{ \times }}\,{\rm{1}}{{\rm{0}}^{{\rm{23}}}}\,{\rm{Cl}}\,{\rm{atoms}}\]

 

And here is how we can combine the conversions into a one-step process:

 

\[{\rm{N}}\,{\rm{(PC}}{{\rm{l}}_{\rm{5}}}{\rm{)}}\,{\rm{ = }}\,{\rm{54}}{\rm{.0}}\,\cancel{{{\rm{g}}\,{\rm{PC}}{{\rm{l}}_{\rm{5}}}}}\,{\rm{ \times }}\,\frac{{{\rm{1}}\;\cancel{{{\rm{mol}}\;{\rm{PC}}{{\rm{l}}_{\rm{5}}}}}}}{{{\rm{208}}{\rm{.5}}\;\cancel{{{\rm{g}}\,{\rm{PC}}{{\rm{l}}_{\rm{5}}}}}}}\; \times \;\frac{{{\rm{6}}{\rm{.02}}\;{\rm{ \times }}\,{\rm{1}}{{\rm{0}}^{{\rm{23}}}}\;\cancel{{{\rm{molecules}}\,{\rm{PC}}{{\rm{l}}_{\rm{5}}}}}}}{{{\rm{1}}\;\cancel{{{\rm{mol}}\;{\rm{PC}}{{\rm{l}}_{\rm{5}}}}}}}\,{\rm{ \times }}\,\frac{{{\rm{5}}\,{\rm{atoms}}\,{\rm{of Cl}}}}{{{\rm{1}}\;\cancel{{{\rm{molecule}}\;{\rm{of}}\;{\rm{PC}}{{\rm{l}}_{\rm{5}}}}}}}\;{\rm{ = }}\;{\rm{7}}{\rm{.85}}\,{\rm{ \times }}\,{\rm{1}}{{\rm{0}}^{{\rm{23}}}}\,{\rm{Cl}}\,{\rm{atoms}}\,\]

 

Another example: How many atoms of oxygen are there in a 35.0 g sample of glucose, C6H12O6?

The molar mass of glucose is:

 

M (C6H12O6) = 6 x 12 + 12 x 1.0 + 6 x 16 = 180 g/mol

 

Therefore, the two conversion factors for going mass → moles, and moles → molecules are:

 

\[\frac{{{\rm{1}}\,{\rm{mol}}\,{{\rm{C}}_{\rm{6}}}{{\rm{H}}_{{\rm{12}}}}{{\rm{O}}_{\rm{6}}}}}{{{\rm{180}}{\rm{.}}\,{\rm{g}}}}\;,\,\,\frac{{{\rm{6}}{\rm{.022}}\;{\rm{ \times }}\,{\rm{1}}{{\rm{0}}^{{\rm{23}}}}\;{{\rm{C}}_{\rm{6}}}{{\rm{H}}_{{\rm{12}}}}{{\rm{O}}_{\rm{6}}}\,{\rm{molecules}}}}{{{\rm{1}}\,{\rm{mol}}\,{{\rm{C}}_{\rm{6}}}{{\rm{H}}_{{\rm{12}}}}{{\rm{O}}_{\rm{6}}}}}\]

 

So, we can use these factors to convert the mass to the number of molecules:

 

\[{\rm{N}}\;{\rm{(}}{{\rm{C}}_{\rm{6}}}{{\rm{H}}_{{\rm{12}}}}{{\rm{O}}_{\rm{6}}}{\rm{)}}\,{\rm{ = }}\;{\rm{35}}{\rm{.0}}\cancel{{\rm{g}}}\;{\rm{ \times }}\,\frac{{{\rm{1}}\cancel{{{\rm{mol}}\,{{\rm{C}}_{\rm{6}}}{{\rm{H}}_{{\rm{12}}}}{{\rm{O}}_{\rm{6}}}}}}}{{{\rm{180}}{\rm{.}}\cancel{{\rm{g}}}}}\;{\rm{ \times }}\,\frac{{{\rm{6}}{\rm{.022}}\;{\rm{ \times }}\,{\rm{1}}{{\rm{0}}^{{\rm{23}}}}\;{{\rm{C}}_{\rm{6}}}{{\rm{H}}_{{\rm{12}}}}{{\rm{O}}_{\rm{6}}}\,{\rm{molecules}}}}{{{\rm{1}}\,\cancel{{{\rm{mol}}\,{{\rm{C}}_{\rm{6}}}{{\rm{H}}_{{\rm{12}}}}{{\rm{O}}_{\rm{6}}}}}}}\; = \;1.17\;{\rm{ \times }}\,{\rm{1}}{{\rm{0}}^{{\rm{23}}}}\;\;\;\]

 

To find the number of oxygen atoms, we multiply this number by 6 because there are 6 oxygen atoms in molecules of glucose. Combining all the steps together, we can write the following conversion sequence:

 

\[{\rm{N}}\;{\rm{(O)}}\,{\rm{ = }}\;{\rm{35}}{\rm{.0}}\cancel{{{\rm{g}}\;{{\rm{C}}_{\rm{6}}}{{\rm{H}}_{{\rm{12}}}}{{\rm{O}}_{\rm{6}}}}}\;{\rm{ \times }}\,\frac{{{\rm{1}}\cancel{{{\rm{mol}}\,{{\rm{C}}_{\rm{6}}}{{\rm{H}}_{{\rm{12}}}}{{\rm{O}}_{\rm{6}}}}}}}{{{\rm{180}}{\rm{.}}\cancel{{\rm{g}}}}}\;{\rm{ \times }}\,\frac{{{\rm{6}}{\rm{.022}}\;{\rm{ \times }}\,{\rm{1}}{{\rm{0}}^{{\rm{23}}}}\;\cancel{{{{\rm{C}}_{\rm{6}}}{{\rm{H}}_{{\rm{12}}}}{{\rm{O}}_{\rm{6}}}\,{\rm{molecules}}}}}}{{{\rm{1}}\,\cancel{{{\rm{mol}}\,{{\rm{C}}_{\rm{6}}}{{\rm{H}}_{{\rm{12}}}}{{\rm{O}}_{\rm{6}}}}}}}\; \times \frac{{{\rm{6}}\,{\rm{atoms}}\,{\rm{of O}}}}{{{\rm{1}}\,\cancel{{{{\rm{C}}_{\rm{6}}}{{\rm{H}}_{{\rm{12}}}}{{\rm{O}}_{\rm{6}}}\,{\rm{molecule}}}}}}\, = \;7.02\;{\rm{ \times }}\,{\rm{1}}{{\rm{0}}^{{\rm{23}}}}\,{\rm{atoms}}\,{\rm{of O}}\;\;\;\]

 

 Converting the Number of Atoms to Grams

Another type of question relating the mass and the number of atoms is to determine the mass of a sample given the number of atoms. This is the reverse calculation, and we are going to use the reciprocal of the conversion factors that we used in the previous calculations.  

For example, let’s say we were asked to determine the mass of an iron sample that contains 7.58 x 1024 atoms. These are the conversion factors that we are going to need:

 

 

The first one allows converting the number of atoms to moles, and the second is converting the moles to mass.

 

\[{\rm{7}}{\rm{.58 x 1}}{{\rm{0}}^{{\rm{24}}}}\,\cancel{{{\rm{Fe}}\,{\rm{atoms}}}}\,{\rm{ \times }}\,\frac{{{\rm{1}}\;\cancel{{{\rm{mol}}\;{\rm{Fe}}}}}}{{{\rm{6}}{\rm{.03}}\;{\rm{ \times }}\,{\rm{1}}{{\rm{0}}^{{\rm{23}}}}\;\cancel{{{\rm{Fe}}\;{\rm{atoms}}}}}}\,{\rm{ \times }}\,\frac{{{\rm{55}}{\rm{.8}}\;{\rm{g}}\,{\rm{Fe}}}}{{{\rm{1}}\;\cancel{{{\rm{mol}}\;{\rm{Fe}}}}}}\;{\rm{ = }}\,{\rm{703}}\,{\rm{g}}\;\]

 

Practice

1.

Calculating Moles from Mass

Determine the number of moles in 59.7 grams of Al.

answer

2.21 mol

Solution

The moles and mass are connected through the molar mass. The molar mass of Al is 27.0 g/mol, therefore the conversion factor is:

 

\[\frac{{{\rm{27}}{\rm{.0}}\;{\rm{g}}\,{\rm{Al}}}}{{{\rm{1}}\;{\rm{mol}}}}\]

 

\[{\rm{n}}\;{\rm{(Al)}}\,{\rm{ = }}\;{\rm{59}}{\rm{.7}}\cancel{{{\rm{g}}\,{\rm{Al}}}}\;{\rm{ \times }}\,\frac{{{\rm{1}}\,{\rm{mol}}}}{{{\rm{27}}{\rm{.0}}\,\cancel{{{\rm{g}}\,{\rm{Al}}}}}}\;{\rm{ = }}\;{\rm{2}}{\rm{.21}}\;{\rm{mol}}\]

2.

Determine the number of moles in 2.41 grams of FeO.

answer

0.0336 mol

Solution

The moles and mass are connected through the molar mass. The molar mass of FeO is 71.8 g/mol, therefore the conversion factor is:

 

\[\frac{{{\rm{71}}{\rm{.8}}\;{\rm{g}}\,{\rm{FeO}}}}{{{\rm{1}}\;{\rm{mol}}}}\]

 

\[{\rm{n}}\;{\rm{(FeO)}}\,{\rm{ = }}\;{\rm{2}}{\rm{.41}}\cancel{{{\rm{g}}\,{\rm{FeO}}}}\;{\rm{ \times }}\,\frac{{{\rm{1}}\,{\rm{mol}}}}{{{\rm{71}}{\rm{.8}}\,\cancel{{{\rm{g}}\,{\rm{FeO}}}}}}\;{\rm{ = }}\;{\rm{0}}{\rm{.0336}}\;{\rm{mol}}\]

3.

Calculate the number of moles in 0.647 grams of Al2O3.

answer

0.00634 mol

Solution

The moles and mass are connected through the molar mass. The molar mass of Al2O3 is 102 g/mol, therefore the conversion factor is:

 

\[\frac{{{\rm{102}}\;{\rm{g}}\,{\rm{A}}{{\rm{l}}_{\rm{2}}}{{\rm{O}}_{\rm{3}}}}}{{{\rm{1}}\;{\rm{mol}}}}\]

 

\[{\rm{n}}\;{\rm{(A}}{{\rm{l}}_{\rm{2}}}{{\rm{O}}_{\rm{3}}}{\rm{)}}\,{\rm{ = }}\;{\rm{0}}{\rm{.647}}\cancel{{{\rm{g}}\,{\rm{A}}{{\rm{l}}_{\rm{2}}}{{\rm{O}}_{\rm{3}}}}}\;{\rm{ \times }}\,\frac{{{\rm{1}}\,{\rm{mol}}}}{{{\rm{102}}\,\cancel{{{\rm{g}}\,{\rm{A}}{{\rm{l}}_{\rm{2}}}{{\rm{O}}_{\rm{3}}}}}}}\;{\rm{ = }}\;{\rm{0}}{\rm{.00634}}\;{\rm{mol}}\]

4.

Determine the number of moles in 3.56 grams of Mg(OH)2.

answer

0.0611 mol

Solution

The moles and mass are connected through the molar mass. The molar mass of Mg(OH)2 is 58.3 g/mol, therefore the conversion factor is:

 

\[\frac{{{\rm{58}}{\rm{.3}}\;{\rm{g}}\,{\rm{Mg}}{{\left( {{\rm{OH}}} \right)}_{\rm{2}}}}}{{{\rm{1}}\;{\rm{mol}}}}\]

 

\[{\rm{n}}\;{\rm{(Mg}}{\left( {{\rm{OH}}} \right)_{\rm{2}}}{\rm{)}}\,{\rm{ = }}\;{\rm{3}}{\rm{.56}}\cancel{{{\rm{g}}\,{\rm{Mg}}{{\left( {{\rm{OH}}} \right)}_{\rm{2}}}}}\;{\rm{ \times }}\,\frac{{{\rm{1}}\,{\rm{mol}}}}{{{\rm{58}}{\rm{.3}}\,\cancel{{{\rm{g}}\,{\rm{Mg}}{{\left( {{\rm{OH}}} \right)}_{\rm{2}}}}}}}\;{\rm{ = }}\;{\rm{0}}{\rm{.0611}}\;{\rm{mol}}\]

5.

Determine the number of moles in 0.385 grams of N2O3.

answer

0.00507 mol

Solution

The moles and mass are connected through the molar mass. The molar mass of N2O3 is 76.0 g/mol, therefore the conversion factor is:

 

\[\frac{{{\rm{76}}{\rm{.0}}\;{\rm{g}}\,{{\rm{N}}_{\rm{2}}}{{\rm{O}}_{\rm{3}}}}}{{{\rm{1}}\;{\rm{mol}}}}\]

 

\[{\rm{n}}\;{\rm{(}}{{\rm{N}}_{\rm{2}}}{{\rm{O}}_{\rm{3}}}{\rm{)}}\,{\rm{ = }}\;{\rm{0}}{\rm{.385}}\cancel{{{\rm{g}}\,{{\rm{N}}_{\rm{2}}}{{\rm{O}}_{\rm{3}}}}}\;{\rm{ \times }}\,\frac{{{\rm{1}}\,{\rm{mol}}}}{{{\rm{76}}{\rm{.0}}\,\cancel{{{\rm{g}}\,{{\rm{N}}_{\rm{2}}}{{\rm{O}}_{\rm{3}}}}}}}\;{\rm{ = }}\;{\rm{0}}{\rm{.00507}}\;{\rm{mol}}\]

6.

Determine the number of moles in 165 grams of CaSO4.

answer

1.21 mol

Solution

The moles and mass are connected through the molar mass. The molar mass of CaSO4 is 136.1 g/mol, therefore the conversion factor is:

 

\[\frac{{{\rm{136}}{\rm{.1}}\;{\rm{g}}\,{\rm{CaS}}{{\rm{O}}_{\rm{4}}}}}{{{\rm{1}}\;{\rm{mol}}}}\]

 

\[{\rm{n}}\;{\rm{(CaS}}{{\rm{O}}_{\rm{4}}}{\rm{)}}\,{\rm{ = }}\;{\rm{165}}\cancel{{{\rm{g}}\,{\rm{CaS}}{{\rm{O}}_{\rm{4}}}}}\;{\rm{ \times }}\,\frac{{{\rm{1}}\,{\rm{mol}}}}{{{\rm{136}}{\rm{.1}}\,\cancel{{{\rm{g}}\,{\rm{CaS}}{{\rm{O}}_{\rm{4}}}}}}}\;{\rm{ = }}\;{\rm{1}}{\rm{.21}}\;{\rm{mol}}\]

 

7.

Calculate the molar mass of N2O4 and determine how many moles of it are in a 23.9 g sample.

answer

0.260 mol

Solution

The moles and mass are connected through the molar mass. The molar mass of N2O4 is 92.0 g/mol, therefore the conversion factor is:

 

\[\frac{{{\rm{92}}{\rm{.0}}\;{\rm{g}}\,{{\rm{N}}_{\rm{2}}}{{\rm{O}}_{\rm{4}}}}}{{{\rm{1}}\;{\rm{mol}}}}\]

 

\[{\rm{n}}\;{\rm{(}}{{\rm{N}}_{\rm{2}}}{{\rm{O}}_{\rm{4}}}{\rm{)}}\,{\rm{ = }}\;{\rm{23}}{\rm{.9}}\cancel{{{\rm{g}}\,{{\rm{N}}_{\rm{2}}}{{\rm{O}}_{\rm{4}}}}}\;{\rm{ \times }}\,\frac{{{\rm{1}}\,{\rm{mol}}}}{{{\rm{92}}{\rm{.0}}\,\cancel{{{\rm{g}}\,{{\rm{N}}_{\rm{2}}}{{\rm{O}}_{\rm{4}}}}}}}\;{\rm{ = }}\;{\rm{0}}{\rm{.260}}\;{\rm{mol}}\]

8.

Calculate the number of moles in 165 grams of C3H6O.

answer

2.84 mol

Solution

The moles and mass are connected through the molar mass. The molar mass of C3H6O is 58.1 g/mol, therefore the conversion factor is:

 

\[\frac{{{\rm{58}}{\rm{.1}}\;{\rm{g}}\,{{\rm{C}}_{\rm{3}}}{{\rm{H}}_{\rm{6}}}{\rm{O}}}}{{{\rm{1}}\;{\rm{mol}}}}\]

 

\[{\rm{n}}\;{\rm{(}}{{\rm{C}}_{\rm{3}}}{{\rm{H}}_{\rm{6}}}{\rm{O)}}\,{\rm{ = }}\;{\rm{165}}\cancel{{{\rm{g}}\,{{\rm{C}}_{\rm{3}}}{{\rm{H}}_{\rm{6}}}{\rm{O}}}}\;{\rm{ \times }}\,\frac{{{\rm{1}}\,{\rm{mol}}}}{{{\rm{58}}{\rm{.1}}\,\cancel{{{\rm{g}}\,{{\rm{C}}_{\rm{3}}}{{\rm{H}}_{\rm{6}}}{\rm{O}}}}}}\;{\rm{ = }}\;{\rm{2}}{\rm{.84}}\;{\rm{mol}}\]

9.

Determine the number of moles in 452 grams of Co(NO3)3.

answer

1.84 mol

Solution

The moles and mass are connected through the molar mass. The molar mass of Co(NO3)3 is 245 g/mol, therefore the conversion factor is:

 

\[\frac{{{\rm{245}}\;{\rm{g}}\,{\rm{Co}}{{\left( {{\rm{N}}{{\rm{O}}_{\rm{3}}}} \right)}_{\rm{3}}}}}{{{\rm{1}}\;{\rm{mol}}}}\]

 

\[{\rm{n}}\;{\rm{(Co}}{\left( {{\rm{N}}{{\rm{O}}_{\rm{3}}}} \right)_{\rm{3}}}{\rm{)}}\,{\rm{ = }}\;{\rm{452}}\cancel{{{\rm{g}}\,{\rm{Co}}{{\left( {{\rm{N}}{{\rm{O}}_{\rm{3}}}} \right)}_{\rm{3}}}}}\;{\rm{ \times }}\,\frac{{{\rm{1}}\,{\rm{mol}}}}{{{\rm{245}}\,\cancel{{{\rm{g}}\,{\rm{Co}}{{\left( {{\rm{N}}{{\rm{O}}_{\rm{3}}}} \right)}_{\rm{3}}}}}}}\;{\rm{ = }}\;{\rm{1}}{\rm{.84}}\;{\rm{mol}}\]

10.

Calculating Mass from Moles 

Calculate the mass in grams of 0.598 moles of Fe.

answer

33.4 g

Solution

The molar mass of Fe is 55.8 g/mol, therefore the conversion factor is:

 

\[\frac{{{\rm{55}}{\rm{.8}}\;{\rm{g}}\,{\rm{Fe}}}}{{{\rm{1}}\;{\rm{mol}}}}\]

 

\[{\rm{m}}\;{\rm{(Fe)}}\,{\rm{ = }}\;{\rm{0}}{\rm{.598}}\;\cancel{{{\rm{mol}}\,{\rm{Fe}}}}\,{\rm{ \times }}\,\frac{{{\rm{55}}{\rm{.8}}\;{\rm{g}}\,{\rm{Fe}}}}{{{\rm{1}}\;\cancel{{{\rm{mol}}\;{\rm{Fe}}}}}}\;{\rm{ = }}\;{\rm{33}}{\rm{.4}}\;{\rm{g}}\]

11.

Calculate the mass in grams of 0.168 moles of NO.

answer

5.04 g

Solution

The molar mass of NO is 30.0 g/mol, therefore the conversion factor is:

 

\[\frac{{{\rm{30}}{\rm{.0}}\;{\rm{g}}\,{\rm{NO}}}}{{{\rm{1}}\;{\rm{mol}}}}\]

 

\[{\rm{m}}\;{\rm{(NO)}}\,{\rm{ = }}\;{\rm{0}}{\rm{.168}}\;\cancel{{{\rm{mol}}\,{\rm{NO}}}}\,{\rm{ \times }}\,\frac{{{\rm{30}}{\rm{.0}}\;{\rm{g}}}}{{{\rm{1}}\;\cancel{{{\rm{mol}}\;{\rm{NO}}}}}}\;{\rm{ = }}\;{\rm{5}}{\rm{.04}}\;{\rm{g}}\]

 

12.

Calculate the mass in grams of 0.987 moles of (NH4)2S.

answer

67.3 g

Solution

The molar mass of (NH4)2S is 68.2 g/mol, therefore the conversion factor is:

 

\[\frac{{{\rm{68}}{\rm{.2}}\;{\rm{g}}\,{{\left( {{\rm{N}}{{\rm{H}}_{\rm{4}}}} \right)}_{\rm{2}}}{\rm{S}}}}{{{\rm{1}}\;{\rm{mol}}}}\]

\[{\rm{m}}\;{\rm{(}}{\left( {{\rm{N}}{{\rm{H}}_{\rm{4}}}} \right)_{\rm{2}}}{\rm{S)}}\,{\rm{ = }}\;{\rm{0}}{\rm{.987}}\;\cancel{{{\rm{mol}}\,{{\left( {{\rm{N}}{{\rm{H}}_{\rm{4}}}} \right)}_{\rm{2}}}{\rm{S}}}}\,{\rm{ \times }}\,\frac{{{\rm{68}}{\rm{.2}}\;{\rm{g}}}}{{{\rm{1}}\;\cancel{{{\rm{mol}}\;{{\left( {{\rm{N}}{{\rm{H}}_{\rm{4}}}} \right)}_{\rm{2}}}{\rm{S}}}}}}\;{\rm{ = }}\;{\rm{67}}{\rm{.3}}\;{\rm{g}}\]

13.

Calculate the mass in grams of 6.81 moles of Al2(SO4)3.

answer

2.33 x 103 g

Solution

The molar mass of Al2(SO4)3 is 342.2 g/mol, therefore the conversion factor is:

 

\[\frac{{{\rm{342}}{\rm{.2}}\;{\rm{g}}\,{\rm{A}}{{\rm{l}}_{\rm{2}}}{{\left( {{\rm{S}}{{\rm{O}}_{\rm{4}}}} \right)}_{\rm{3}}}}}{{{\rm{1}}\;{\rm{mol}}}}\]

\[{\rm{m}}\;{\rm{(A}}{{\rm{l}}_{\rm{2}}}{\left( {{\rm{S}}{{\rm{O}}_{\rm{4}}}} \right)_{\rm{3}}}{\rm{)}}\,{\rm{ = }}\;{\rm{6}}{\rm{.81}}\;\cancel{{{\rm{mol}}\,{\rm{A}}{{\rm{l}}_{\rm{2}}}{{\left( {{\rm{S}}{{\rm{O}}_{\rm{4}}}} \right)}_{\rm{3}}}}}\,{\rm{ \times }}\,\frac{{{\rm{342}}{\rm{.2}}\;{\rm{g}}}}{{{\rm{1}}\;\cancel{{{\rm{mol}}\;{\rm{A}}{{\rm{l}}_{\rm{2}}}{{\left( {{\rm{S}}{{\rm{O}}_{\rm{4}}}} \right)}_{\rm{3}}}}}}}\;{\rm{ = }}\;{\rm{2}}{\rm{.33}}\, \times \;{10^3}\;{\rm{g}}\]

14.

Calculate the mass in grams of 2.64 moles of methanol, CH3OH.

answer

84.5 g

Solution

The molar mass of CH3OH is 32.0 g/mol, therefore the conversion factor is:

 

\[\frac{{{\rm{32}}{\rm{.0}}\;{\rm{g}}\,{\rm{C}}{{\rm{H}}_{\rm{3}}}{\rm{OH}}}}{{{\rm{1}}\;{\rm{mol}}}}\]

\[{\rm{m}}\;{\rm{(C}}{{\rm{H}}_{\rm{3}}}{\rm{OH)}}\,{\rm{ = }}\;{\rm{2}}{\rm{.64}}\;\cancel{{{\rm{mol}}\,{\rm{C}}{{\rm{H}}_{\rm{3}}}{\rm{OH}}}}\,{\rm{ \times }}\,\frac{{{\rm{32}}{\rm{.0}}\;{\rm{g}}}}{{{\rm{1}}\;\cancel{{{\rm{mol}}\;{\rm{C}}{{\rm{H}}_{\rm{3}}}{\rm{OH}}}}}}\;{\rm{ = }}\;{\rm{84}}{\rm{.5}}\;{\rm{g}}\]

15.

Calculate the mass in grams of 9.42 moles of NiCl2·6H2O.

answer

2.24 x 103 g

Solution

The molar mass of NiCl2·6H2O is129.6 g/mol. Do not forget to add the mass of 6 moles of H2O (6 x 18 = 108 g/mol) to the molar mass of NiCl2. Therefore, the conversion factor is:

 

\[\frac{{{\rm{237}}{\rm{.6}}\;{\rm{g}}\,{\rm{NiC}}{{\rm{l}}_{\rm{2}}}{\rm{\cdot6}}{{\rm{H}}_{\rm{2}}}{\rm{O}}}}{{{\rm{1}}\;{\rm{mol}}}}\]

 

\[{\rm{m}}\;{\rm{(NiC}}{{\rm{l}}_{\rm{2}}}{\rm{\cdot6}}{{\rm{H}}_{\rm{2}}}{\rm{O)}}\,{\rm{ = }}\;{\rm{9}}{\rm{.42}}\;\cancel{{{\rm{mol}}\,{\rm{NiC}}{{\rm{l}}_{\rm{2}}}{\rm{\cdot6}}{{\rm{H}}_{\rm{2}}}{\rm{O}}}}\,{\rm{ \times }}\,\frac{{{\rm{237}}{\rm{.6}}\;{\rm{g}}}}{{{\rm{1}}\;\cancel{{{\rm{mol}}\;{\rm{NiC}}{{\rm{l}}_{\rm{2}}}{\rm{\cdot6}}{{\rm{H}}_{\rm{2}}}{\rm{O}}}}}}\;{\rm{ = }}\;{\rm{2}}{\rm{.24}}\, \times \,{\rm{1}}{{\rm{0}}^{\rm{3}}}\;{\rm{g}}\]

16.

Calculating the Number of Molecules from the Moles

How many molecules are there in a 0.487 mol sample of PCl5?

answer

2.93 x 1023

Solution

To determine the number of molecules, we use the Avogadro’s number which shows the number of particles (molecules) in one mole of the sample.

 

\[\frac{{{\rm{6}}{\rm{.02}}\;{\rm{ \times }}\,{\rm{1}}{{\rm{0}}^{{\rm{23}}}}\;{\rm{molecules}}}}{{{\rm{1}}\,{\rm{mol}}}}\,\,or\,\,\frac{{{\rm{1}}\,{\rm{mol}}}}{{{\rm{6}}{\rm{.02}}\;{\rm{ \times }}\,{\rm{1}}{{\rm{0}}^{{\rm{23}}}}\;{\rm{molecules}}}}\;\;\;\;\]

 

\[{\rm{N}}\;{\rm{(PC}}{{\rm{l}}_{\rm{5}}}{\rm{)}}\,{\rm{ = }}\;{\rm{0}}{\rm{.487}}\,\cancel{{{\rm{mol}}\,{\rm{PC}}{{\rm{l}}_{\rm{5}}}}}\;{\rm{ \times }}\,\frac{{{\rm{6}}{\rm{.022}}\;{\rm{ \times }}\,{\rm{1}}{{\rm{0}}^{{\rm{23}}}}\;{\rm{PC}}{{\rm{l}}_{\rm{5}}}\,{\rm{molecules}}}}{{{\rm{1}}\,\cancel{{{\rm{mol}}\,{\rm{PC}}{{\rm{l}}_{\rm{5}}}}}}}\; = \;2.93\;{\rm{ \times }}\,{\rm{1}}{{\rm{0}}^{{\rm{23}}}}\;\;\;\]

17.

How many molecules (formula units) are there in a 5.84 mol sample of Na2SO3.

answer

3.52 x 1024

Solution

\[{\rm{N}}\;{\rm{(N}}{{\rm{a}}_{\rm{2}}}{\rm{S}}{{\rm{O}}_{\rm{3}}}{\rm{)}}\,{\rm{ = }}\;{\rm{5}}{\rm{.84}}\,\cancel{{{\rm{mol}}\,{\rm{N}}{{\rm{a}}_{\rm{2}}}{\rm{S}}{{\rm{O}}_{\rm{3}}}}}\;{\rm{ \times }}\,\frac{{{\rm{6}}{\rm{.022}}\;{\rm{ \times }}\,{\rm{1}}{{\rm{0}}^{{\rm{23}}}}\;{\rm{N}}{{\rm{a}}_{\rm{2}}}{\rm{S}}{{\rm{O}}_{\rm{3}}}\,{\rm{molecules}}}}{{{\rm{1}}\,\cancel{{{\rm{mol}}\,{\rm{N}}{{\rm{a}}_{\rm{2}}}{\rm{S}}{{\rm{O}}_{\rm{3}}}}}}}\; = \;3.52\;{\rm{ \times }}\,{\rm{1}}{{\rm{0}}^{{\rm{24}}}}\;\;\;\]

18.

How many molecules of sucrose, C12H22O11 are there in a 0.684 mol sample?

answer

4.12 x 1023

Solution

\[{\rm{N}}\;{\rm{(}}{{\rm{C}}_{{\rm{12}}}}{{\rm{H}}_{{\rm{22}}}}{{\rm{O}}_{{\rm{11}}}}{\rm{)}}\,{\rm{ = }}\;{\rm{0}}{\rm{.684}}\,\cancel{{{\rm{mol}}\,{{\rm{C}}_{{\rm{12}}}}{{\rm{H}}_{{\rm{22}}}}{{\rm{O}}_{{\rm{11}}}}}}\;{\rm{ \times }}\,\frac{{{\rm{6}}{\rm{.022}}\;{\rm{ \times }}\,{\rm{1}}{{\rm{0}}^{{\rm{23}}}}\;{{\rm{C}}_{{\rm{12}}}}{{\rm{H}}_{{\rm{22}}}}{{\rm{O}}_{{\rm{11}}}}\,{\rm{molecules}}}}{{{\rm{1}}\,\cancel{{{\rm{mol}}\,{{\rm{C}}_{{\rm{12}}}}{{\rm{H}}_{{\rm{22}}}}{{\rm{O}}_{{\rm{11}}}}}}}}\; = \;4.12\;{\rm{ \times }}\,{\rm{1}}{{\rm{0}}^{{\rm{23}}}}\;\;\;\]

19.

Calculate the number of molecules in a 3.25-mol sample of propane, C3H8.

answer

1.96 x 1024

Solution

\[{\rm{N}}\;{\rm{(}}{{\rm{C}}_{\rm{3}}}{{\rm{H}}_{\rm{8}}}{\rm{)}}\,{\rm{ = }}\;{\rm{3}}{\rm{.25}}\,\cancel{{{\rm{mol}}\,{{\rm{C}}_{\rm{3}}}{{\rm{H}}_{\rm{8}}}}}\;{\rm{ \times }}\,\frac{{{\rm{6}}{\rm{.022}}\;{\rm{ \times }}\,{\rm{1}}{{\rm{0}}^{{\rm{23}}}}\;{{\rm{C}}_{\rm{3}}}{{\rm{H}}_{\rm{8}}}\,{\rm{molecules}}}}{{{\rm{1}}\,\cancel{{{\rm{mol}}\,{{\rm{C}}_{\rm{3}}}{{\rm{H}}_{\rm{8}}}}}}}\; = \;1.96\;{\rm{ \times }}\,{\rm{1}}{{\rm{0}}^{{\rm{24}}}}\;\;\;\]

20.

How many moles is 5.80 x 1025 molecules of POCl3?

answer

96.3 moles

Solution

This is a conversion in reverse directions, therefore, we use the other conversion factor linking the number of moles and number of molecules.

\[{\rm{n}}\;{\rm{(POC}}{{\rm{l}}_{\rm{3}}}{\rm{)}}\,{\rm{ = }}\;5.80\; \times \;{10^{25}}\,\cancel{{{\rm{molecules}}\,{\rm{POC}}{{\rm{l}}_{\rm{3}}}\,}}\;{\rm{ \times }}\,\frac{{{\rm{1}}\,{\rm{mol}}\,{\rm{POC}}{{\rm{l}}_{\rm{3}}}{\rm{ }}}}{{{\rm{6}}{\rm{.022}}\;{\rm{ \times }}\,{\rm{1}}{{\rm{0}}^{{\rm{23}}}}\,\cancel{{{\rm{molecules}}\;{\rm{POC}}{{\rm{l}}_{\rm{3}}}}}}}\; = \;96.3\,{\rm{moles}}\;\;\;\]

21.

Calculating the Number of Molecules from the Mass

How many molecules are there in a 5.12-g sample of K2O?

answer

3.27 x 1022

Solution

There is an extra step here which is to first convert the mass to moles. After this, it is the same conversion as we have seen in the previous section for converting moles to the number of molecules using the Avogadro’s number. You can solve these types of problems by step-by-step or one-step conversion.

 

The first step is to convert the mass to moles:

 

\[{\rm{n}}\;{\rm{(}}{{\rm{K}}_{\rm{2}}}{\rm{O)}}\,{\rm{ = }}\;{\rm{5}}{\rm{.12}}\cancel{{\rm{g}}}\;{\rm{ \times }}\,\frac{{{\rm{1}}\cancel{{{\rm{mol}}}}{{\rm{K}}_{\rm{2}}}{\rm{O}}}}{{{\rm{94}}{\rm{.2}}\cancel{{\rm{g}}}}}\;{\rm{ = }}\;{\rm{0}}{\rm{.0543524}}\;{\rm{mol}}\;\]

 

Keep the decimals and round them off in the last step.

Once we have the number of moles, we can calculate the number of molecules.

 

\[{\rm{N}}\;{\rm{(}}{{\rm{K}}_{\rm{2}}}{\rm{O)}}\,{\rm{ = }}\;{\rm{0}}{\rm{.0543524}}\,\cancel{{{\rm{mol}}\,{{\rm{K}}_{\rm{2}}}{\rm{O}}}}\;{\rm{ \times }}\,\frac{{{\rm{6}}{\rm{.022}}\;{\rm{ \times }}\,{\rm{1}}{{\rm{0}}^{{\rm{23}}}}\;{{\rm{K}}_{\rm{2}}}{\rm{O}}\,{\rm{molecules}}}}{{{\rm{1}}\,\cancel{{{\rm{mol}}\,{{\rm{K}}_{\rm{2}}}{\rm{O}}}}}}\; = \;3.27\;{\rm{ \times }}\,{\rm{1}}{{\rm{0}}^{{\rm{22}}}}\;\;\;\]

 

A quicker method is to combine these two steps in one. Just make sure to use the correct conversion factor that allows canceling the units.

 

\[{\rm{N}}\;{\rm{(}}{{\rm{K}}_{\rm{2}}}{\rm{O)}}\,{\rm{ = }}\;{\rm{5}}{\rm{.12}}\cancel{{\rm{g}}}\;{\rm{ \times }}\,\frac{{{\rm{1}}\cancel{{{\rm{mol}}\,{{\rm{K}}_{\rm{2}}}{\rm{O}}}}}}{{{\rm{94}}{\rm{.2}}\cancel{{\rm{g}}}}}\;{\rm{ \times }}\,\frac{{{\rm{6}}{\rm{.022}}\;{\rm{ \times }}\,{\rm{1}}{{\rm{0}}^{{\rm{23}}}}\;{{\rm{K}}_{\rm{2}}}{\rm{O}}\,{\rm{molecules}}}}{{{\rm{1}}\,\cancel{{{\rm{mol}}\,{{\rm{K}}_{\rm{2}}}{\rm{O}}}}}}\; = \;3.27\;{\rm{ \times }}\,{\rm{1}}{{\rm{0}}^{{\rm{22}}}}\;\;\;\]

22.

How many molecules of glucose, C6H12O6 are there in a 35.0 g sample?

answer

1.17 x 1023

Solution

Refer to the first problem in “Calculating the number of molecules from the mass” for a detailed explanation.

 

\[{\rm{N}}\;{\rm{(}}{{\rm{C}}_{\rm{6}}}{{\rm{H}}_{{\rm{12}}}}{{\rm{O}}_{\rm{6}}}{\rm{)}}\,{\rm{ = }}\;{\rm{35}}{\rm{.0}}\cancel{{\rm{g}}}\;{\rm{ \times }}\,\frac{{{\rm{1}}\cancel{{{\rm{mol}}\,{{\rm{C}}_{\rm{6}}}{{\rm{H}}_{{\rm{12}}}}{{\rm{O}}_{\rm{6}}}}}}}{{{\rm{180}}{\rm{.2}}\cancel{{\rm{g}}}}}\;{\rm{ \times }}\,\frac{{{\rm{6}}{\rm{.022}}\;{\rm{ \times }}\,{\rm{1}}{{\rm{0}}^{{\rm{23}}}}\;{{\rm{C}}_{\rm{6}}}{{\rm{H}}_{{\rm{12}}}}{{\rm{O}}_{\rm{6}}}\,{\rm{molecules}}}}{{{\rm{1}}\,\cancel{{{\rm{mol}}\,{{\rm{C}}_{\rm{6}}}{{\rm{H}}_{{\rm{12}}}}{{\rm{O}}_{\rm{6}}}}}}}\; = \;1.17\;{\rm{ \times }}\,{\rm{1}}{{\rm{0}}^{{\rm{23}}}}\;\;\;\]

23.

Calculate the number of molecules of butane, C4H10, in its 2.40-gram sample.

answer

2.49 x 1022

Solution

\[{\rm{N}}\;{\rm{(}}{{\rm{C}}_{\rm{4}}}{{\rm{H}}_{{\rm{10}}}}{\rm{)}}\,{\rm{ = }}\;{\rm{2}}{\rm{.40}}\cancel{{\rm{g}}}\;{\rm{ \times }}\,\frac{{{\rm{1}}\cancel{{{\rm{mol}}\,{{\rm{C}}_{\rm{4}}}{{\rm{H}}_{{\rm{10}}}}}}}}{{{\rm{58}}{\rm{.1}}\cancel{{\rm{g}}}}}\;{\rm{ \times }}\,\frac{{{\rm{6}}{\rm{.022}}\;{\rm{ \times }}\,{\rm{1}}{{\rm{0}}^{{\rm{23}}}}\;{{\rm{C}}_{\rm{4}}}{{\rm{H}}_{{\rm{10}}}}\,{\rm{molecules}}}}{{{\rm{1}}\,\cancel{{{\rm{mol}}\,{{\rm{C}}_{\rm{4}}}{{\rm{H}}_{{\rm{10}}}}}}}}\; = \;2.49\;{\rm{ \times }}\,{\rm{1}}{{\rm{0}}^{{\rm{22}}}}\;\;\;\]

24.

How many Ethylene, C2H4 molecules are present in a 46.2 g sample? The molar mass of C2H4 is 28.1 g/mol.

answer

9.90 x 1023

Solution

\[{\rm{N}}\;{\rm{(}}{{\rm{C}}_{\rm{2}}}{{\rm{H}}_{\rm{4}}}{\rm{)}}\,{\rm{ = }}\;{\rm{46}}{\rm{.2}}\cancel{{\rm{g}}}\;{\rm{ \times }}\,\frac{{{\rm{1}}\cancel{{{\rm{mol}}\,{{\rm{C}}_{\rm{2}}}{{\rm{H}}_{\rm{4}}}}}}}{{{\rm{28}}{\rm{.1}}\cancel{{\rm{g}}}}}\;{\rm{ \times }}\,\frac{{{\rm{6}}{\rm{.022}}\;{\rm{ \times }}\,{\rm{1}}{{\rm{0}}^{{\rm{23}}}}\;{{\rm{C}}_{\rm{2}}}{{\rm{H}}_{\rm{4}}}\,{\rm{molecules}}}}{{{\rm{1}}\,\cancel{{{\rm{mol}}\,{{\rm{C}}_{\rm{2}}}{{\rm{H}}_{\rm{4}}}}}}}\; = \;9.90\;{\rm{ \times }}\,{\rm{1}}{{\rm{0}}^{{\rm{23}}}}\;\;\;\]

25.

Calculating the Number of Atoms

Calculate the number of atoms in a 2.56-g sample of Ca.

answer

3.84 x 1022

Solution

We have seen how to convert the number of moles to the number of molecules using the Avogadros’ number. Finding the number of atoms follows the same steps when working with atoms. Because one mole contains 6.022 x 1023 atoms of Ca, we can calculate the number of atoms by multiplying the number of moles by the Avogadro’s number:

 

\[{\rm{N}}\;{\rm{(Ca)}}\,{\rm{ = }}\;{\rm{2}}{\rm{.56}}\cancel{{\rm{g}}}\;{\rm{ \times }}\,\frac{{{\rm{1}}\cancel{{{\rm{mol}}\,{\rm{Ca}}}}}}{{{\rm{40}}{\rm{.1}}\cancel{{\rm{g}}}}}\;{\rm{ \times }}\,\frac{{{\rm{6}}{\rm{.022}}\;{\rm{ \times }}\,{\rm{1}}{{\rm{0}}^{{\rm{23}}}}\;{\rm{Ca}}\,{\rm{atoms}}}}{{{\rm{1}}\,\cancel{{{\rm{mol}}\,{\rm{Ca}}}}}}\; = \;3.84\;{\rm{ \times }}\,{\rm{1}}{{\rm{0}}^{{\rm{22}}}}\;\;\;\]

26.

How many carbon atoms are there in a 0.590 mol sample of CCl4.

answer

3.55 x 1023

Solution

To find the number of atoms/ions in a molecule, multiply the number of molecules by the subscript of that atom. In this case, the subscript of carbon is one, so the number of carbon atoms is going to be equal to the number of molecules.

 

\[{\rm{N}}\;{\rm{(C atoms)}}\,{\rm{ = }}\;{\rm{0}}{\rm{.590}}\,\cancel{{{\rm{mol}}\,{\rm{CC}}{{\rm{l}}_{\rm{4}}}}}\;{\rm{ \times }}\,\frac{{{\rm{6}}{\rm{.022}}\;{\rm{ \times }}\,{\rm{1}}{{\rm{0}}^{{\rm{23}}}}\;\cancel{{{\rm{CC}}{{\rm{l}}_{\rm{4}}}\,{\rm{molecules}}}}}}{{{\rm{1}}\,\cancel{{{\rm{mol}}\,{\rm{CC}}{{\rm{l}}_{\rm{4}}}}}}}\;{\rm{ \times }}\,\frac{{{\rm{1}}\,{\rm{atom}}\,{\rm{of}}\,{\rm{C}}}}{{{\rm{1}}\,\cancel{{{\rm{CC}}{{\rm{l}}_{\rm{4}}}\,{\rm{molecule}}}}\,}}\; = \;3.55\;{\rm{ \times }}\,{\rm{1}}{{\rm{0}}^{{\rm{23}}}}\;\;\;\]

27.

How many carbon atoms are there in a 0.964 mol sample of C2H6.

answer

1.16 x 1024

Solution

This is very similar to the previous example with the difference that there are 2 carbon atoms in each molecule of C2H6. Therefore, there is going to be twice more carbon atoms than C2H6 molecules.

 

\[{\rm{N}}\;{\rm{(C atoms)}}\,{\rm{ = }}\;{\rm{0}}{\rm{.964}}\,\cancel{{{\rm{mol}}\,{{\rm{C}}_{\rm{2}}}{{\rm{H}}_{\rm{6}}}}}\;{\rm{ \times }}\,\frac{{{\rm{6}}{\rm{.022}}\;{\rm{ \times }}\,{\rm{1}}{{\rm{0}}^{{\rm{23}}}}\;\cancel{{{{\rm{C}}_{\rm{2}}}{{\rm{H}}_{\rm{6}}}\,{\rm{molecules}}}}}}{{{\rm{1}}\,\cancel{{{\rm{mol}}\,{{\rm{C}}_{\rm{2}}}{{\rm{H}}_{\rm{6}}}}}}}\;{\rm{ \times }}\,\frac{{{\rm{2}}\,{\rm{atom}}\,{\rm{of}}\,{\rm{C}}}}{{{\rm{1}}\,\cancel{{{{\rm{C}}_{\rm{2}}}{{\rm{H}}_{\rm{6}}}\,{\rm{molecule}}}}\,}}\; = \;1.16\;{\rm{ \times }}\,{\rm{1}}{{\rm{0}}^{{\rm{24}}}}\;\;\;\]

 

So, if we stop the conversion before the last step, we’d see that there are 5.805 x 1023 molecules of C2H6 and therefore, we multiply that number by two to determine the number of carbon atoms.

28.

Which sample contains a more Cl atoms: a) 1.25 moles of CH2Cl2 b) 2.15 moles of CH3Cl

answer

a) 1.25 moles of CH2Cl2

Solution

Sometimes, you may need to save time and solve the problem using a shortcut. For example, here, if we figure which sample has a greater number of moles of Cl, we can conclude that it also has more Cl atoms because we only multiply both numbers by the Avogadros’ number.

 

So, 1.25 mol CH2Cl2 contains 2 x 1.25 = 2.50 mol Cl atoms. 2.15 mol CH3Cl, on the other hand, contains the same number of Cl atoms because each molecule contains one atom of Cl.

Therefore,  1.25 mol CH2Cl2 contains more Cl atoms than 2.15 mol CH3Cl.

 

Let’s confirm this with a complete calculation to practice it a little more too:

 

\[{\rm{N}}\;{\rm{(Cl atoms)}}\,{\rm{ = }}\;{\rm{1}}{\rm{.25}}\,\cancel{{{\rm{mol}}\,{\rm{C}}{{\rm{H}}_{\rm{2}}}{\rm{C}}{{\rm{l}}_{\rm{2}}}}}\;{\rm{ \times }}\,\frac{{{\rm{6}}{\rm{.022}}\;{\rm{ \times }}\,{\rm{1}}{{\rm{0}}^{{\rm{23}}}}\;\cancel{{{\rm{C}}{{\rm{H}}_{\rm{2}}}{\rm{C}}{{\rm{l}}_{\rm{2}}}\,{\rm{molecules}}}}}}{{{\rm{1}}\,\cancel{{{\rm{mol}}\,{\rm{C}}{{\rm{H}}_{\rm{2}}}{\rm{C}}{{\rm{l}}_{\rm{2}}}}}}}\;{\rm{ \times }}\,\frac{{{\rm{2}}\,{\rm{atom}}\,{\rm{of}}\,{\rm{Cl}}}}{{{\rm{1}}\,\cancel{{{\rm{C}}{{\rm{H}}_{\rm{2}}}{\rm{C}}{{\rm{l}}_{\rm{2}}}\,{\rm{molecule}}}}\,}}\; = \;1.51\;{\rm{ \times }}\,{\rm{1}}{{\rm{0}}^{{\rm{24}}}}\;\;\;\]

 

\[{\rm{N}}\;{\rm{(Cl atoms)}}\,{\rm{ = }}\;{\rm{2}}{\rm{.15}}\,\cancel{{{\rm{mol}}\,{\rm{C}}{{\rm{H}}_{\rm{3}}}{\rm{Cl}}}}\;{\rm{ \times }}\,\frac{{{\rm{6}}{\rm{.022}}\;{\rm{ \times }}\,{\rm{1}}{{\rm{0}}^{{\rm{23}}}}\;\cancel{{{\rm{C}}{{\rm{H}}_{\rm{3}}}{\rm{Cl}}\,{\rm{molecules}}}}}}{{{\rm{1}}\,\cancel{{{\rm{mol}}\,{\rm{C}}{{\rm{H}}_{\rm{3}}}{\rm{Cl}}}}}}\;{\rm{ \times }}\,\frac{{{\rm{1}}\,{\rm{atom}}\,{\rm{of}}\,{\rm{Cl}}}}{{{\rm{1}}\,\cancel{{{\rm{C}}{{\rm{H}}_{\rm{3}}}{\rm{Cl}}\,{\rm{molecule}}}}\,}}\; = \;1.29\;{\rm{ \times }}\,{\rm{1}}{{\rm{0}}^{{\rm{24}}}}\;\;\;\]

 

29.

The molecular formula of morphine is C17H19NO3. How many carbon atoms are in a 34.7-gram sample of morphine?

 

answer

1.25 x 1024

Solution

One molecule of C17H19NO3 contains 17 carbon atoms, so once we find the number of molecules, we multiply it by 17:

 

\[{\rm{N}}\;{\rm{(C}}\,{\rm{atoms)}}\,{\rm{ = }}\;{\rm{34}}{\rm{.7}}\;\cancel{{{\rm{g}}\;{\rm{Mor}}{\rm{.}}\,}}{\rm{ \times }}\,\frac{{{\rm{1}}\cancel{{{\rm{mol}}\,{\rm{Mor}}{\rm{.}}}}}}{{{\rm{285}}{\rm{.3}}\,\cancel{{{\rm{g}}\,{\rm{Mor}}{\rm{.}}}}}}\;{\rm{ \times }}\,\frac{{{\rm{6}}{\rm{.022}}\;{\rm{ \times }}\,{\rm{1}}{{\rm{0}}^{{\rm{23}}}}\;\cancel{{{\rm{Mor}}{\rm{.}}\,{\rm{molecules}}}}}}{{{\rm{1}}\,\cancel{{{\rm{mol}}\,{\rm{Mor}}{\rm{.}}}}}}\;{\rm{ \times }}\,\frac{{{\rm{17}}\;{\rm{C}}\,{\rm{atoms}}}}{{{\rm{1}}\,\cancel{{{\rm{molecule}}\,{\rm{Mor}}{\rm{.}}}}}}{\rm{ = }}\;{\rm{1}}{\rm{.25}}\;{\rm{ \times }}\,{\rm{1}}{{\rm{0}}^{{\rm{24}}}}\;\;\;\]

30.

Isopropyl alcohol, also known as isopropanol, has found a widespread application in the preparation of pharmaceutical products. Answer the following questions considering that the molecular formula of isopropanol is C3H8O.

a) How many moles of C3H8O are contained in a 12.0 g sample of the alcohol?
b) How many molecules of C3H8O are contained in a 12.0 g sample of the alcohol?
c) How many atoms of oxygen are contained in a 12.0 g sample of the isopropyl alcohol (C3H8O)?
d) How many atoms of carbon are contained in a 12.0 g sample of the isopropyl alcohol (C3H8O)?

a)
answer

a) 0.200 moles of C3H8O

b)
answer

b) 1.20 x 1023 molecules of C3H8O

c)
answer

c) 1.20 x 1023 oxygen atoms

d)
answer

d) 3.60 x 1023 carbon atoms

video

31.

Check Also

Leave a Comment

1686018142