When discussing the molar solubility and solubility product constant (Ksp), we mentioned that the values pertain to saturated solutions of the given compound.
For example, the molar solubility of BaOS4 is 3.87 x 10-5 mol/L. Therefore, it starts precipitating only once the concentration goes higher than 3.87 x 10-5 mol/L which means below this concentration, it is dissociated to ions. The solubility product, Ksp for BaOS4 is 1.5 x 10-9:
BaSO4(s) ⇆ Ba2+(aq) + SO42-(aq)
Ksp = [Ba2+][ SO42-]
So, let’s say we want to find out if there will be any precipitation in a solution containing 2.0 x 10-6 M Ba2+ and 2.0 x 10-6 M SO42-.
To do this, we use the ion product quotient, Q which is the same expression as Ksp only that in this case, we use the initial concentrations of the ions. Conceptually, this is no different than the reaction quotient we discussed in chemical equilibrium.
So, Q is calculated for any concentrations of the ions, while the Ksp is the value specifically given for the concentrations at equilibrium when Q = Ksp.
BaSO4(s) ⇆ Ba2+(aq) + SO42-(aq)
Q = [Ba2+]0[SO42-]0
Where [Ba2+]0 and [SO42-]0 are the initial concentrations of the ions. And by “initial” we mean as soon as the ions appear in the solution. The threshold for precipitation to start is when Q becomes larger than Ksp. Q > Ksp indicates there is simply too much of the ions floating around and some of them must react and precipitate from the solution. On the other hand, if Q < Ksp, this means there is not enough of the ions and the solution can handle (dissolve) more, therefore, there will be no precipitation.
To make it more visual, we can write the Q and Ksp correlation with the expression and the specific value.
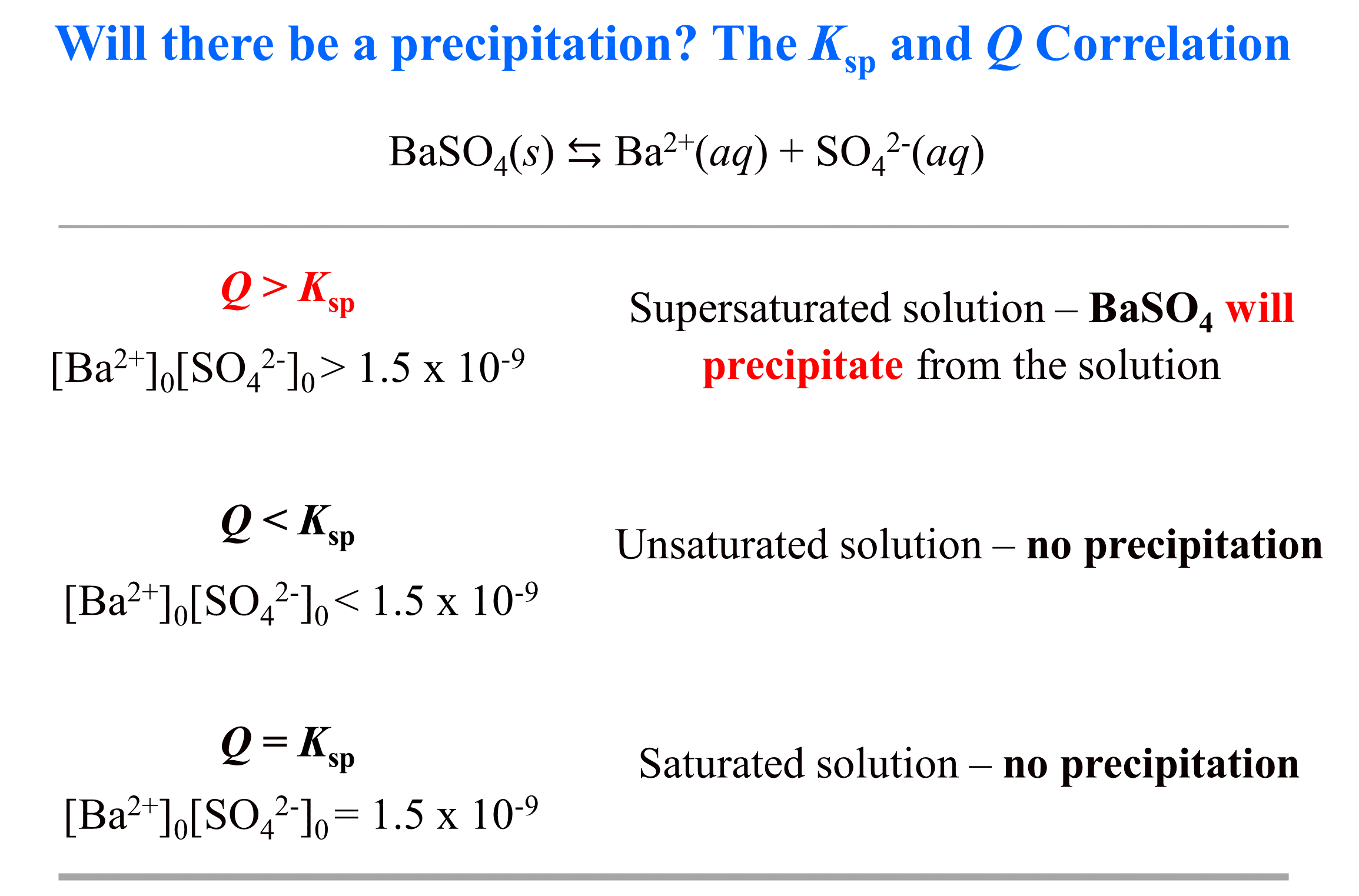
Notice that in the discussion above, we have not mentioned where the Ba2+ and SO42- ions coming from. You cannot have a solution with a separate anion or a cation, and neither can you store them separated in one solution because, as we mentioned, at some point they will react and precipitate.
Therefore, the principle of Q and Ksp correlation is used to determine if there will be a precipitation when certain amounts of two solutions are mixed.
For example,
Will there be any precipitation observed if 30.0 mL of 0.10 M Ba(NO3)2 solution are added to 50.0 mL of 0.10 M Na2SO4? Ksp for BaSO4 is 1.5 × 10−9
The first part is to identify the possible precipitate if not evident from the problem. In this case, you can see it because of the Ksp value, and because we talked about it already. However, let’s say the precipice wasn’t evident. So, you need to write the reaction between the two compounds:
Ba(NO3)2(aq) + Na2SO4(aq) → BaSO4(s) + 2NaNO3(aq)
Based on the solubility rules, the only precipitate that can form here is BaSO4, so go ahead and write the expression for Ksp and the corresponding dissociation equation:
BaSO4(s) ⇆ Ba2+(aq) + SO42–(aq)
Ksp = [Ba2+][SO42–]
Next, determine the concentration of the two compounds once the solutions are mixed. Remember, to add up the volumes of the two solutions:
V = 30.0 + 50.0 = 80.0 mL = 0.0800 L
You can either determine the concentration of the salt in the final solution using the dilution equation or first calculate the moles and then divide it by the volume of the final solution. Let’s go with the first approach and let V2 be the volume of the final solution.
Assigning V1 as the volume of the initial 30.0 mL (0.0300 L) solution of For Ba(NO3)2, we can calculate its concentration the final solution (M2) using the equation for dilution:
M1V1 = M2V2
0.100 mol/L x 0.0300 L = 0.0800 L x M2
\[{{\rm{M}}_{\rm{2}}}\;{\rm{ = }}\;\frac{{{\rm{0}}{\rm{.100 mol/L \times 0}}{\rm{.0300 L}}}}{{{\rm{0}}{\rm{.0800 L}}}}\, = \,0.0375\;M\]
For Na2SO4, let this be the V2, and the V1 is the initial 50.0 mL (0.0500 L). Using the equation for dilution, the concentration in the new solution (M2) will be:
M1V1 = M2V2
0.100 mol/L x 0.0500 L = 0.0800 L x M2
\[{{\rm{M}}_{\rm{2}}}\;{\rm{ = }}\;\frac{{{\rm{0}}{\rm{.100 mol/L \times 0}}{\rm{.0500 L}}}}{{{\rm{0}}{\rm{.0800 L}}}}\, = \,0.0625\;M\]
Next, determine the concentration of Ba2+ and SO42– ions based on the stoichiometric ratio of the ions with the salt. For this, you can write the corresponding dissociation equations:
Ba(NO3)2(aq) → Ba2+(aq) + 2NO3–(aq)
Na2SO4(aq) → 2Na+(aq) + SO42-(aq)
Because of the 1:1 ratio, there will be 0.0375 M Ba2+ and 0.0625 M SO42- ions when the solutions are mixed.
At this point, calculate the quotient using the initial concentrations, [Ba2+]0 and [ SO42–]0 of the ions before any precipitation occurs:
BaSO4(s) ⇆ Ba2+(aq) + Ba2+(aq)
Q = [Ba2+]0[ SO42–]0 = (0.0375) (0.0625) = 0.00306 = 2.34 x 10-3
Q > Ksp, therefore, BaSO4 will form as a precipitate
Let’s do another example when the given concentration of ions does not cause precipitation.
Will a precipitate form when 100. mL of 0.035 M Pb(NO3)2 is added to 100. mL of 0.045 M NaCl? Ksp for PbCl2 is 1.6 × 10−5.
First, identify the possible precipitate if not given. In this case, you can see because of the Ksp value, however, let’s say the precipice wasn’t evident. Write the reaction between the two compounds:
Pb(NO3)2(aq) + 2NaCl(aq) → PbCl2(s) + 2NaNO3(aq)
Based on the solubility rules, the only precipitate that can form here is PbCl2, so go ahead and write the expression for Ksp and the corresponding dissociation equation:
PbCl2(s) ⇆ Pb2+(aq) + 2Cl–(aq)
Ksp = [Pb2+][Cl–]2
Next, determine the concentration of the two compounds once the solutions are mixed. Remember, to add up the volumes of the two solutions for the total volume:
V = 100. + 100. = 200. mL = 0.200 L
Let’s, this time, calculate the moles first.
n (Pb(NO3)2) = 0.100 L x 0.035 M = 0.0035 mol
n (NaCl) = 0.100 L x 0.045 M = 0.0045 mol
The concentrations in the new solution will then be:
M (Pb(NO3)2) = 0.0035 mol ÷ 0.200 L = 0.0175 M
M (NaCl) = 0.0045 mol ÷ 0.200 L = 0.0225 M
Next, determine the concentration of Pb2+ and Cl– ions based on the stoichiometric ratio of the ions with the salt. For this, you can write the corresponding dissociation equations:
Pb(NO3)2(aq) → Pb2+(aq) + 2NO3–(aq)
NaCl(aq) → Na+(aq) + Cl–(aq)
Because of the 1:1 ratio, there will be 0.0175 M Pb2+ and 0.0225 M Cl– ions when the solutions are mixed.
At this point, calculate the quotient using the initial concentrations [Pb2+]0 and [ Cl–]0 of the ions before any precipitation occurs:
PbCl2(s) ⇆ Pb2+(aq) + 2Cl–(aq)
Q = [Pb2+]0[Cl–]02= (0.0175) (0.0225)2 = 8.9 x 10-6
Q is smaller than Ksp, therefore, no precipitate will form.
Check Also
- Buffer Solutions
- The Henderson–Hasselbalch Equation
- The pH of a Buffer Solution
- Preparing a Buffer with a Specific pH
- The Common Ion Effect
- The pH and pKa Relationship
- Strong Acid–Strong Base Titrations
- Titration of a Weak Acid by a Strong Base
- Titration of a Weak Base by a Strong Acid
- Titration of Polyprotic Acids
- Buffer Solutions Practice Problems
- Ksp and Molar Solubility
- The Effect of a Common Ion on Solubility
- The Effect of pH on Solubility
- Ksp and Molar Solubility Practice Problems
