The VSEPR (valence shell electron pair repulsion) theory predicts the geometry of atoms or molecules. It is based on the principle that atoms and lone pairs repel each other because of electrostatic forces and thus, they are positioned as far away as possible to give the molecule an optimal geometry. To determine the geometry, we count the sum of the number of atoms and lone pairs around the central atom. This number is called the electron groups (EG) or steric number (SN) and it defines the geometry of the molecule.
Steric Number
For example, let’s determine the steric number of Be in BeCl2. The first thing you need to do is draw the Lewis structure including all the lone pairs of electrons if there are any. Here are the summary steps for drawing Lewis structures, and if you need to review it, go over the article here.
1. Write the correct skeletal structure for the molecule.
* Hydrogen atoms are always terminal (only one bond)
* Put more electronegative elements in terminal positions
2. Sum the valence electrons from all the atoms.
3. Use a pair of electrons to form a bond between each pair of bound atoms.
4. Add the remaining electrons to satisfy the octet for more electronegative atom first.
5. If any atoms lack an octet, make a double or triple bond to give them an octet.
So, the first step is to draw the correct skeletal structure. Beryllium is the central atom and, therefore, the structure would be:
![]()
There is a total of 2 + 2×7 = 16 valence electrons, and we use four of them to make the bonds. The remaining 12 go on the Cl atoms as lone pairs:
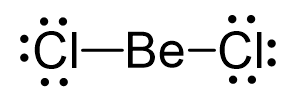
Because the Be has only two atoms and no lone pairs, its steric number is two. Notice that we do not count the lone pairs on the Cl atoms, since we are determining the geometry of the central atom. Another important thing you need to remember is that it does not matter whether the central atom is connected with single, double, or triple bonds – we only count the number of atoms and lone pairs it bears.
For example, the steric number of carbon in HCN is still two even though it is connected with the nitrogen via a triple bond:
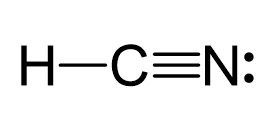
So, once again, keep in mind that multiple bonds do not matter – we treat them as single bonds and only count the atoms and lone pairs for the electron group/steric number.
Let’s put a little chart of example for steric number ranging from 2-6:
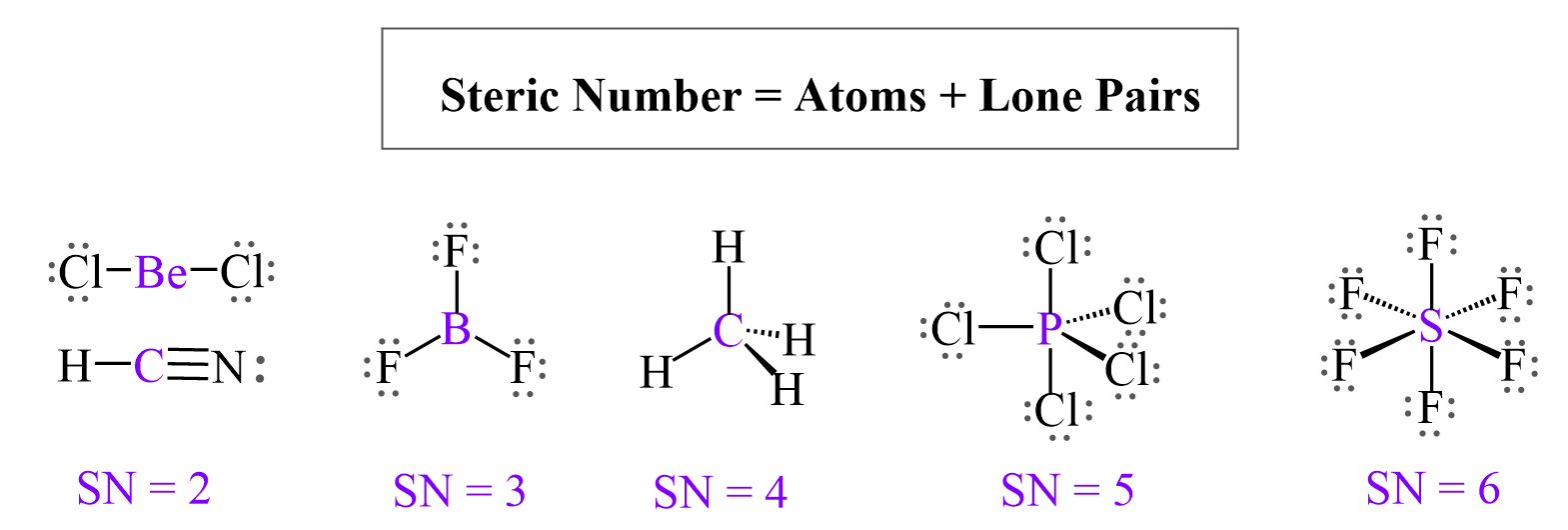
Now, it is not always that the central atom has no lone pairs, and we only need to worry about the number of atoms. For example, the oxygen in water has two atoms (hydrogens) connected to it, but when determining is geometry, we need to also consider the effect of the two lone pairs:
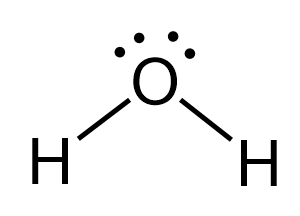
To make this easier, we can classify the compounds into two groups: the ones with lone pairs on the central atoms, and the ones without them.
Central Atoms with no Lone Pair(s)
For central atoms with no lone pairs, there are 5 molecular geometries and approximate bond angles you need to know.
So, let’s discuss these geometries in a little more detail.
Two Atoms – Linear Geometry
We have seen how the BeCl2 adopts a linear geometry because of the two Cl atoms on the Be. Another example is carbon dioxide, CO2:

This molecule also has a linear geometry because the carbon has only two atoms and no lone pairs on it.
Three Atoms – Trigonal Planar Geometry
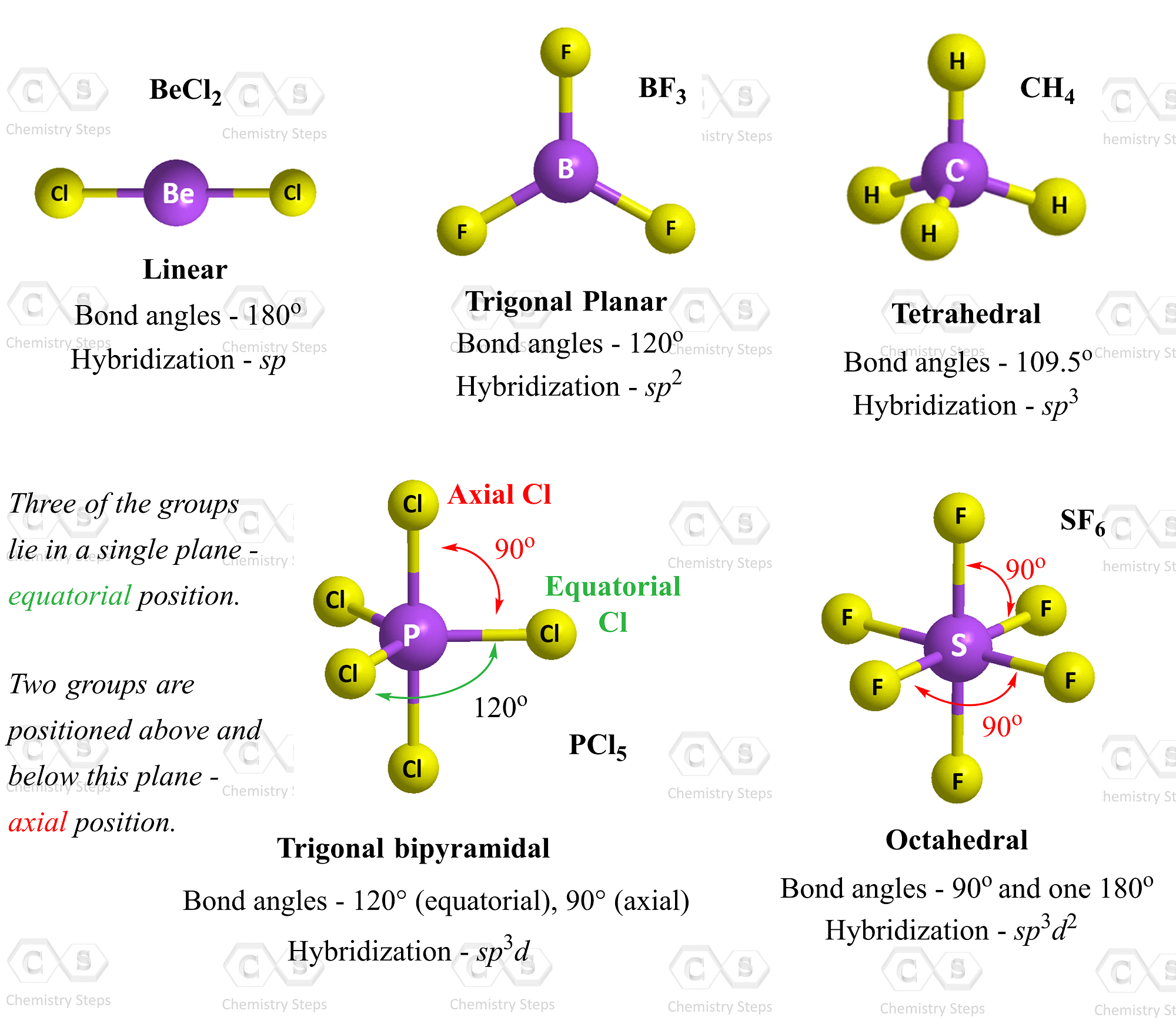
Boron is the central atom, so we can draw the skeletal structure as:
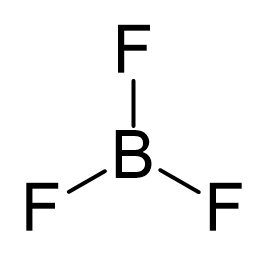
There is a total of 3 + 3 x 7 = 24 electrons, and halogens on terminal positions are always going to have 3 lone pairs of electrons. Therefore, 3 x 6 = 18 electrons on the fluorenes, and the remaining 6 are used to make the three covalent bonds:
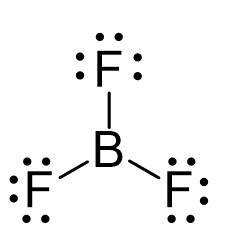
The central atom has three bonds and no lone pairs, therefore, both the electron and molecular geometries are trigonal planar. The bond angle between the fluorine atoms is 120o.
The steric number (the sum of the number of the atoms and lone pairs) of B is 3 which corresponds to sp2-hybridization.
Four Atoms – Tetrahedral Geometry
The most common example of an atom with four atoms and no lone pairs is methane. CH4.

This is the Lewis structure of methane, and one may expect that the bond angles should be 90o. However, it turns out that there is a geometry that allows for a more optimal arrangement of four atoms, and it is called tetrahedral geometry. The advantage is that the bond angle between the groups is 109.5o unlike the 90o we see in the Lewis structure.

Five Atoms – Trigonal Bipyramidal Geometry
The best arrangement for five groups around the central unit is achieved with trigonal bipyramidal geometry. An example of trigonal bipyramidal geometry is the molecule PCl5:
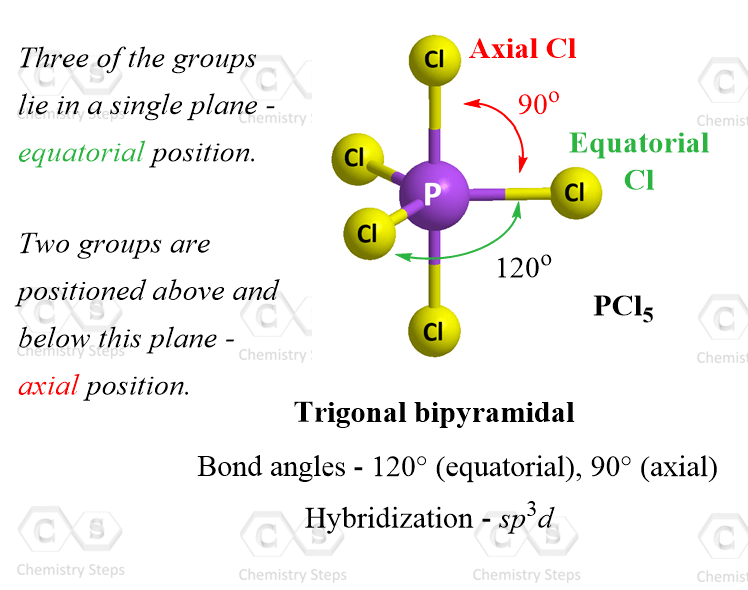
Notice that not all the angles in the trigonal bipyramidal structure are same. This is because the geometry is not as symmetrical, and we have two positions: equatorial and axial. From this view, the equatorial positions are pointing to the sides and the angles between the equatorial positions are 120°. The axial positions are on the bottom and the top. The angle between these two is 180o whereas the angle between the axial and equatorial positions is 90°.
Six Atoms – Octahedral Geometry
When six atoms are connected to a central atom, it adopts what is called octahedral geometry. A good example in general chemistry is sulfur hexafluoride, SF6:
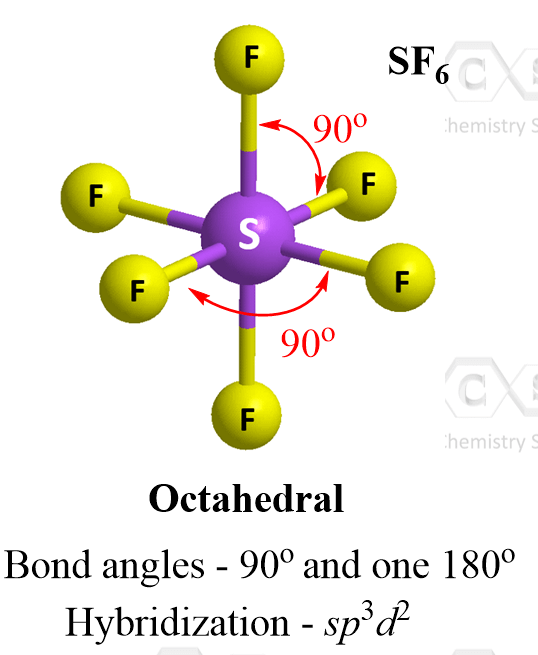
Four of the groups lie in a single plane, and the other two are above and below it. The angles between all neighboring groups 90° while the ones separated with an atom is 180o.
Molecular and Electron Geometry
Before we get to the geometry of molecules with lone pair(s), you need to know that a structure is characterized by molecular and electron geometry, which sometimes may be the same. So, when is the “sometimes” you may ask? For all the examples, where the central atom has no lone pair, the electron and molecular geometries are the same.
However, the presence of a lone pair(s) affects the geometry and therefore, the naming strategy of the molecule. For example, the electron geometry of ammonia, NH3 is tetrahedral while the molecular geometry is trigonal pyramidal:
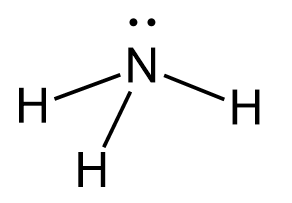
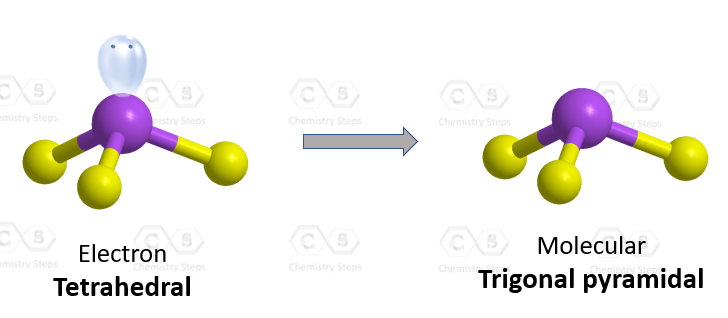
Let’s first see the significance of the lone pair on nitrogen. It is important as if it wasn’t there, we would have a hypothetic molecule with a flat/planar geometry:

Now, for the electron geometry, we do everything as seen before. That is count the number of atoms and lone pairs and name it according to the steric number. For the molecular geometry, we keep the shape as it is, but we ignore the lone pairs. This brings a few new names such as the trigonal pyramidal which we have not seen in the five geometries mentioned earlier.
Why do we ignore the lone pair for naming the molecular geometry? One way to look at it is the fact that electrons are infinitely smaller and lighter than nuclei and when looking on modern microscopes, we don’t see them.
Let’s now go over the examples of structures with lone pairs and see how the names of electron and molecular geometries vary.
Four Electron Groups with Lone Pairs
We just saw the example of ammonia as a molecule with three atoms and one lone pair.

Again, the central atom has 3 atoms and a lone pair (SN = 4). Therefore, the electron geometry is tetrahedral while the molecular geometry is trigonal pyramidal:

It is important to know that a lone pair has a greater repulsion than a bonding pair of electrons (bonding pair means an atom in this context). If all the groups in a tetrahedral geometry are the same, then the angle is 109.5o as we have seen for methane. However, in ammonia, the angle between the hydrogen atoms is smaller, 107o because of the greater repulsion of the lone pair.
Two Atoms and Two Lone Pairs
The example here has to be water:

The central atom has a steric number of 4 – two atoms and two lone pairs, and therefore, the electron geometry, therefore, is tetrahedral, and the molecular geometry is bent.
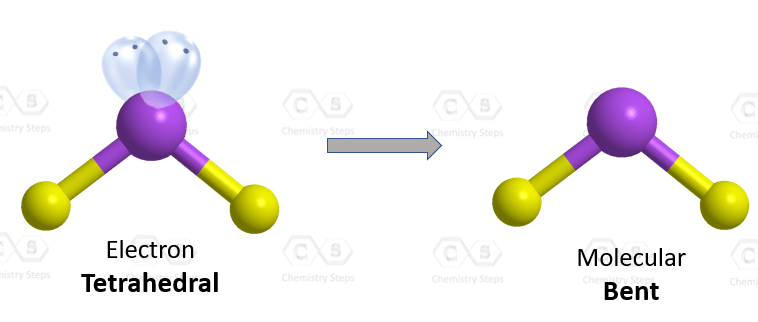
As expected, the two lone pairs make the bond angle between the hydrogen atoms even smaller bringing it down to 104.5o.
Five Electron Groups with Lone Pairs
Four Atoms and One Lone Pair: Let’s consider the Lewis structure of SF4:
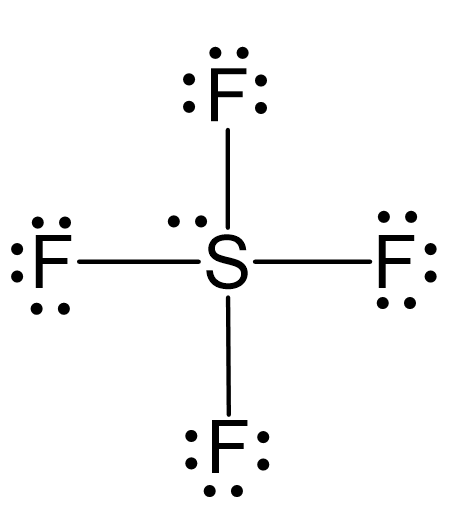
The steric number of sulfur is 4 +1 = 5, and therefore, the electron geometry is trigonal bipyramidal.
To determine the molecular geometry, we need to ignore the lone pair and see what shape is obtained. What we need to know though is where the lone pair is in the first place. Is it in an axial or equatorial position? Because lone pairs exert larger repulsive forces, it is favorable for them to occupy an equatorial position. This puts them at 120o relative to the other equatorial groups and there are only two groups (axial and equatorial) that are at 90o. On the other hand, if the lope pair was in an axial portion, it would have three 90° interactions with bonding pairs.
So, when the lone pair in the equatorial position is disregarded, a molecular geometry is seen which is called a seesaw:
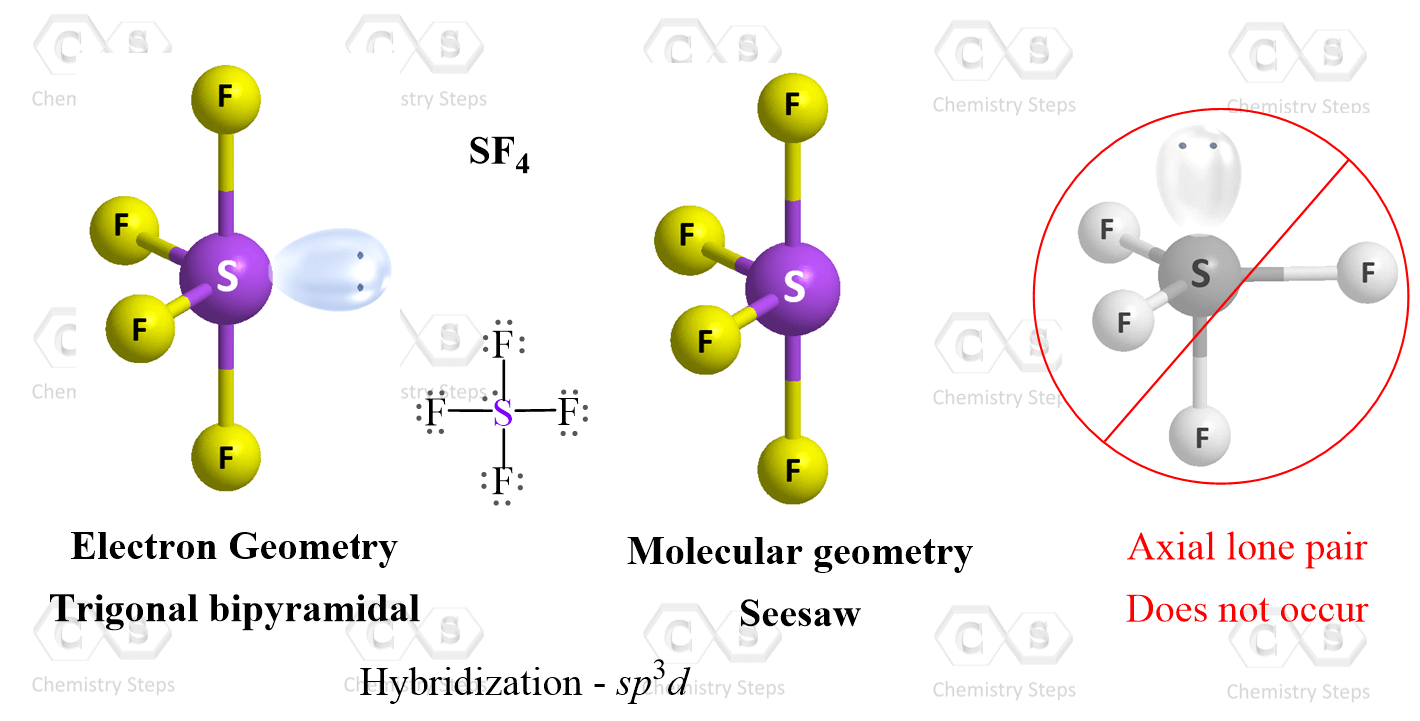
Three Atoms and Two Lone Pairs
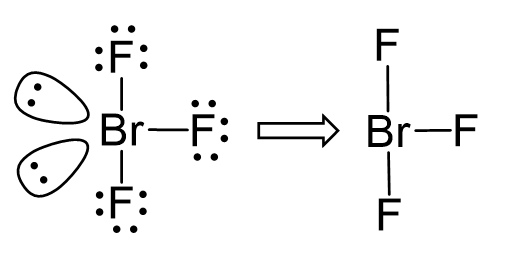
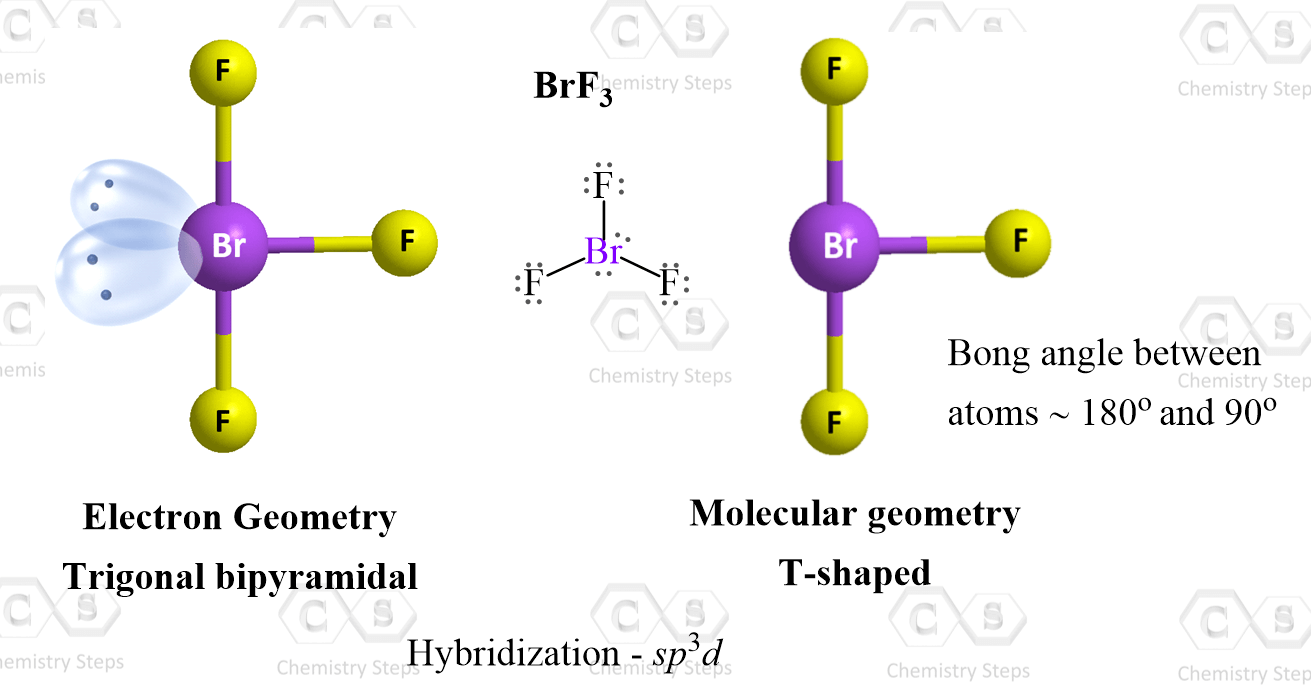
Another example of trigonal bipyramidal electron geometry is BrF3:
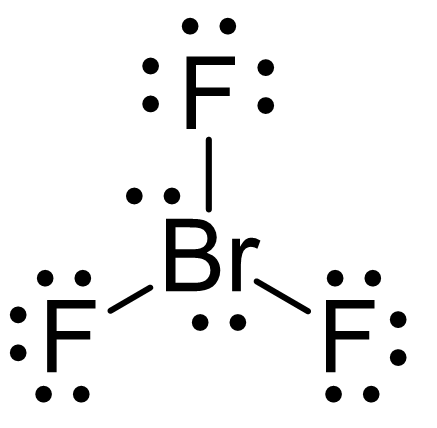
The difference with SF4 is that it has two lone pairs in the equatorial position, and therefore, the molecular geometry is called T-shaped:
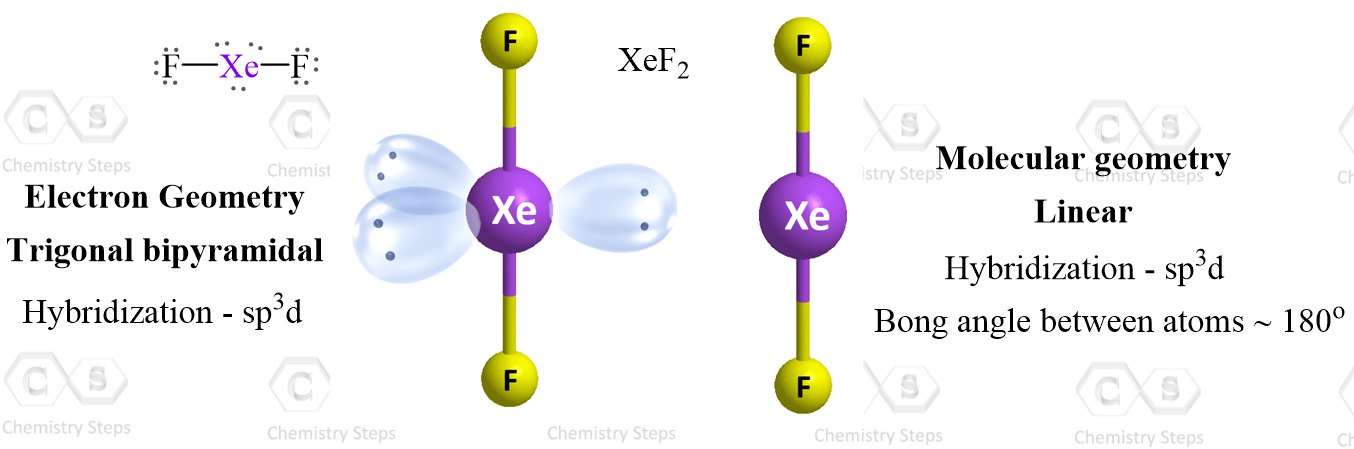
Two Atoms and Three Lone Pairs
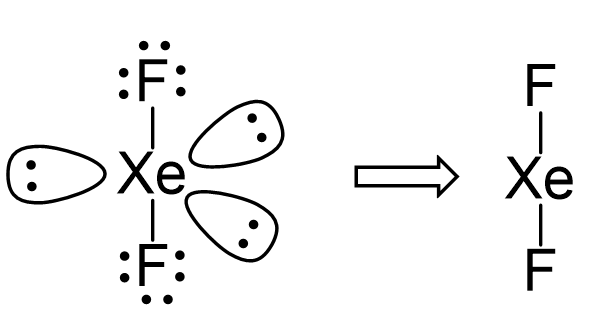
Consider the Lewi structure of XeF2:
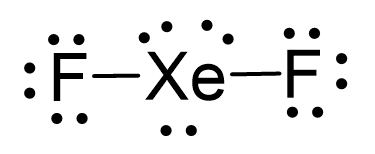
There are 5 units around the central atom, and therefore, the electron geometry is trigonal bipyramidal while the molecular geometry is linear.
Six Electron Groups with Lone Pairs
Two Atoms and Three Lone Pairs:
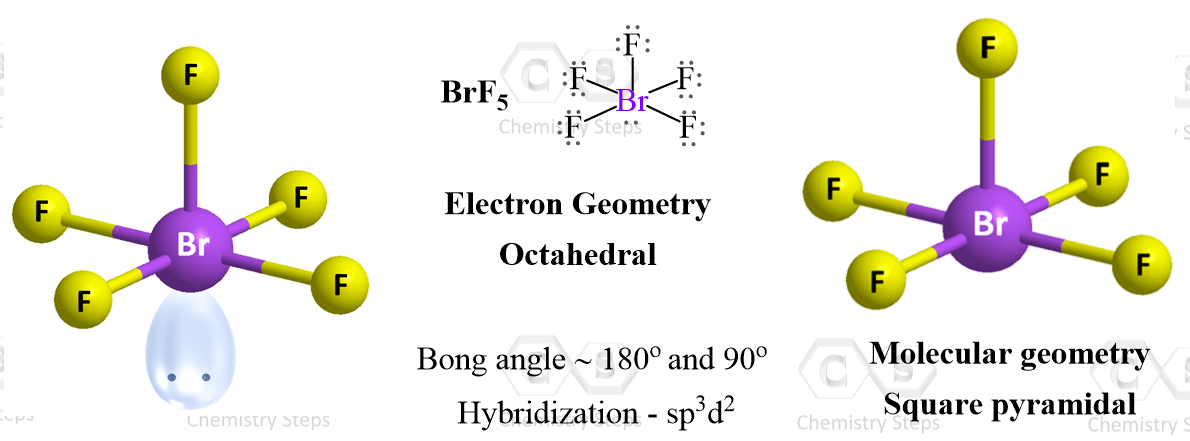
Let’s consider the Lewis structure of BrF5:
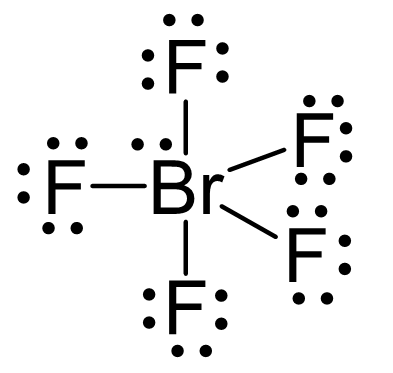
The central atom is bromine which is surrounded by six electron groups – five atoms and one lone pair. Because the steric number is six, the electron geometry, due to the six electron groups, is octahedral. The geometry is symmetrical and therefore, all six positions are equivalent which makes it possible to put the lone pair(s) in any one of these positions.
When there is only one lone pair like in BrF5, the resulting molecular geometry is called square pyramidal.
Four Atoms and Two Lone Pair
Xef4 falls into this category as the central atom has four atoms and two lone pairs:
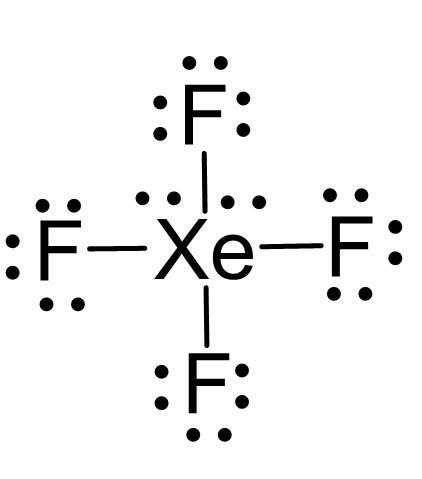
There are 6 units around the central atom, and therefore, the electron geometry is octahedral while the molecular geometry is square planar.
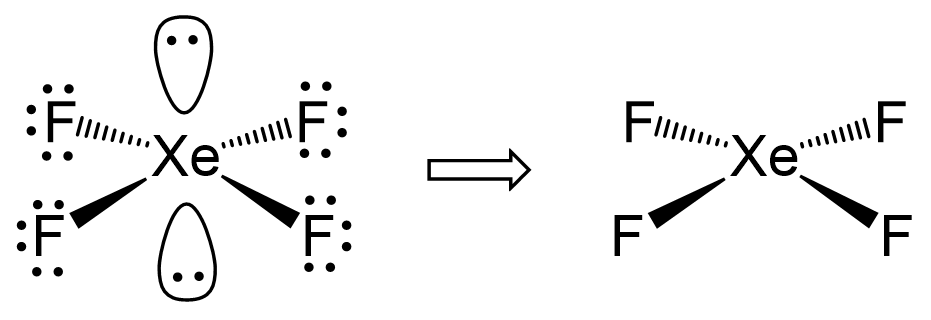
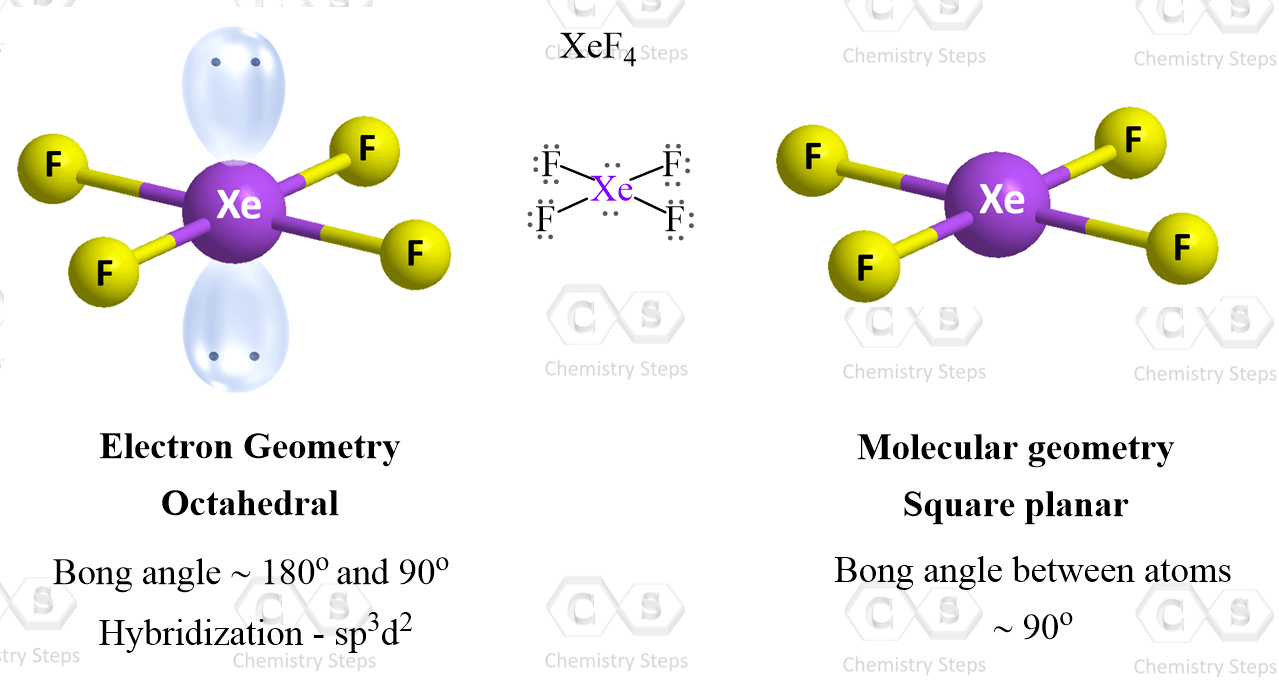
In the end, I would like to mention that there is a separate post on VSEPR theory tailored toward organic molecules. On one hand, it is a little easier since carbon, the main element in organic chemistry, only makes four bonds at most, and therefore, the steric number goes up to 4.
On the other hand, a lot of organic molecules are larger, and studying their structures takes new skill sets.
Practice
Determine the electron geometry and molecular geometry of the following molecules using the VSEPR model.
| (a) BF3 | (b) CH2O | (c) HCN | (d) BeCl2 | (e) CH2Cl2 |
| (f) SOCl2 | (g) SO2 | (h) PCl5 | (i) XeO4 | (j) NCl3 |
| (k) SiCl4 | (l) SF2 | (m) H2S | (n) SO3 | (o) COCl2 |
| (p) PCl3 | (q) OF2 | (r) BrF5 | (s) N2O | (t) SF6 |
| (u) POCl3 | (x) XeF2 | (y) XeF4 | (z) C2H2 |
Determine the electron and molecular geometry of each ion using the VSEPR theory: (a) NH4+, (b) H3O+, (c) CN– (d) SCN–, (e) CO32-, (f) ClO3–, (g) SO42- (h) PO43- (i) SO32– (j) NO2– (k) BF4– (l) NO3–.
| (a) NH4+ | (b) H3O+ | (c) CN– | (d) SCN– | (e) CO32- |
| (f) ClO3– | (g) SO42- | (h) PO43- | (i) SO32– | (j) NO2– |
| (k) BF4– | (l) NO3– |
For each molecular geometry, determine if there are any lone pairs on the central atom and name the molecular and electron geometries accordingly:
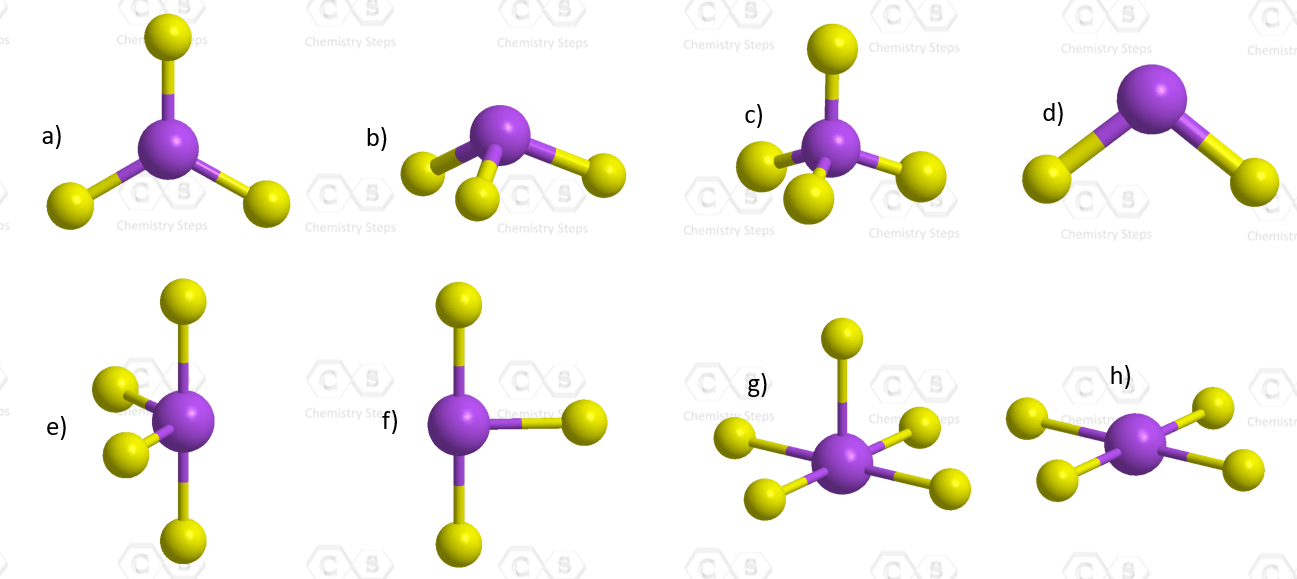
Below is shown the molecular geometry of ReF7. How would you name this geometry and what bond angles would you expect it to have?
For each marked atom, add any missing lone pairs of electrons to determine the steric number, electron and molecular geometry, approximate bond angles, and the hybridization state.
You can also download the questions as a PDF worksheet to print and work on here.
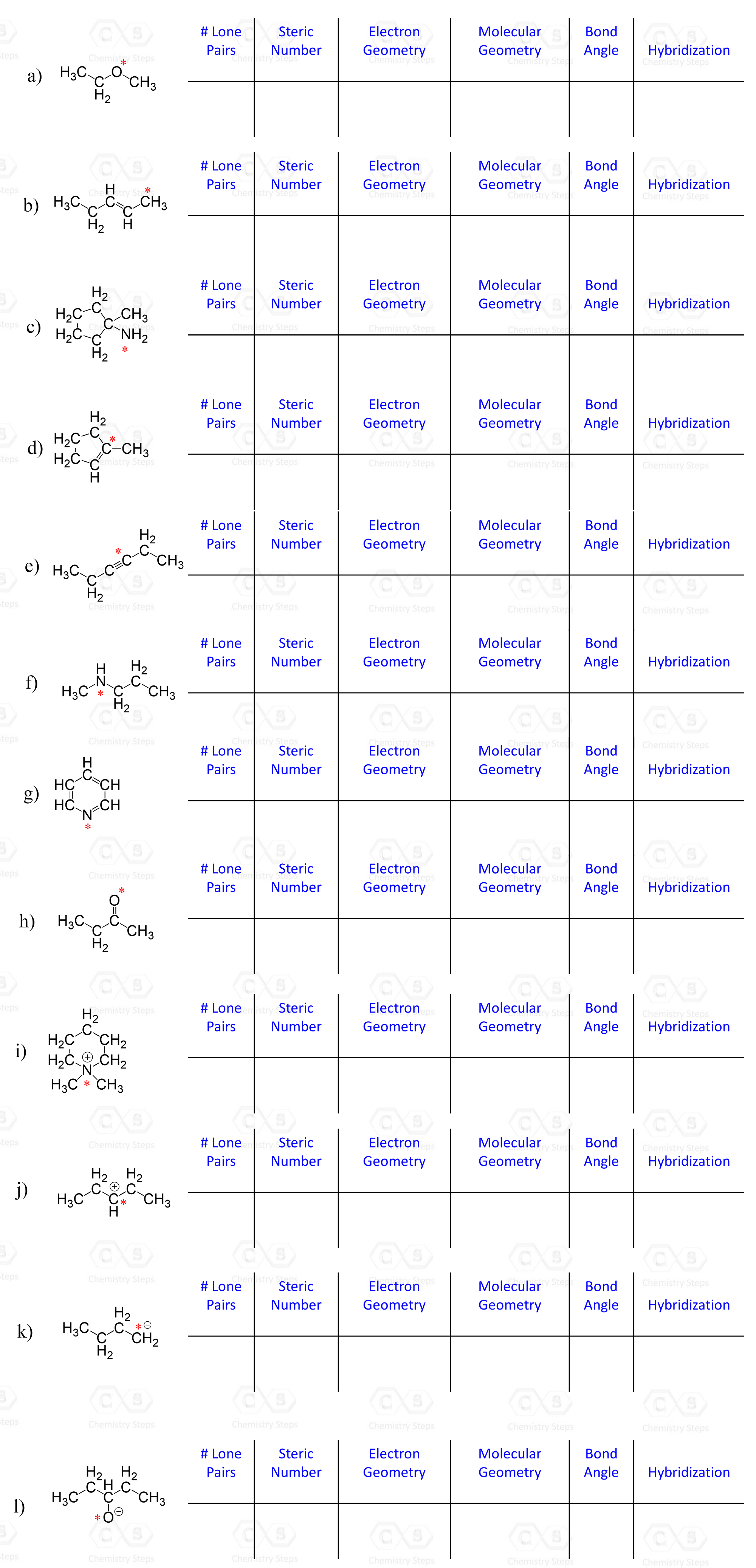
Determine the molecular geometry of each marked atom in the following molecule:

What is the molecular geometry of the nitrogen in the following molecules?

What is the molecular geometry of the nitrogen in the following molecules?

Determine the approximate bond angle in the following molecule:

Determine the approximate bond angle in the following molecule:

The angle between carbon 2 and the lone pair on the nitrogen is:

Check Also
- Lewis Dot Symbols
- The Ionic Bond
- The Covalent Bond
- Sigma and Pi Bonds
- Electronegativity and Bond Polarity
- The Octet Rule
- Formal Charges
- Lewis Structures and the Octet Rule
- Lewis Structures Practice Problems
- Resonance Structures
- VSEPR Theory Practice Problems
- Hybridization of Atomic Orbitals
- sp, sp2, sp3, sp3d, and sp3d2Hybridization Practice Problems

